You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
volume of a barn
- Thread starter eddy2017
- Start date
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
Now back to the other prism. Do you have all the necessary dimensions? If not, can you calculate what's missing?Oh, yes, cubic yards. I should have know that.
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Vt =(area of the triangular base) * (height) , V=Bh (where B is the area of the base)
But we know a formula to find the area of a triangle .
Area of a triangle= ½ bh
So we can plug ½ bh in the place of B in the equation.
Now, let’s rewrite the formula knowing this.
A = 1/2 (bh) h
I am gonna label the two h's differently not to get confused.
A = 1/2 (bh1) h2
h1= the height that I used to find the area of the triangle (so it is the height of the triangle)
h2= it is the h for the original formula (V=Bh) this h stands for the height of the prism.
the next step is to identiy these variables in our prism.
Please, can you confirm if I am going well. Just a like would be enough.
But we know a formula to find the area of a triangle .
Area of a triangle= ½ bh
So we can plug ½ bh in the place of B in the equation.
Now, let’s rewrite the formula knowing this.
A = 1/2 (bh) h
I am gonna label the two h's differently not to get confused.
A = 1/2 (bh1) h2
h1= the height that I used to find the area of the triangle (so it is the height of the triangle)
h2= it is the h for the original formula (V=Bh) this h stands for the height of the prism.
the next step is to identiy these variables in our prism.
Please, can you confirm if I am going well. Just a like would be enough.
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
Looks good.Vt =(area of the triangular base) * (height) , V=Bh (where B is the area of the base)
But we know a formula to find the area of a triangle .
Area of a triangle= ½ bh
So we can plug ½ bh in the place of B in the equation.
Now, let’s rewrite the formula knowing this.
A = 1/2 (bh) h
I am gonna label the two h's differently not to get confused.
A = 1/2 (bh1) h2
h1= the height that I used to find the area of the triangle (so it is the height of the triangle)
h2= it is the h for the original formula (V=Bh) this h stands for the height of the prism.
the next step is to identiy these variables in our prism.
Please, can you confirm if I am going well. Just a like would be enough.
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Great. I continue. TxLooks good.
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Identifying variables.
A = 1/2 (bh1) h2
A= 1/2 (24*
I am stuck here. I am confused about the heights given.
h1 is the height of the triangle, and the problem says that is 9 yd but from base to peak.
If i take the whole 9 yd= or 27 ft I will be taking part of the rectangular prism too. I don't think that is ok, or is it?.
A = 1/2 (bh1) h2
A= 1/2 (24*
I am stuck here. I am confused about the heights given.
h1 is the height of the triangle, and the problem says that is 9 yd but from base to peak.
If i take the whole 9 yd= or 27 ft I will be taking part of the rectangular prism too. I don't think that is ok, or is it?.
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
Yes, this is the missing dimension, but it's possible to calculate it. Look at the drawing of the whole barn and see which dimensions can help you.Identifying variables.
A = 1/2 (bh1) h2
A= 1/2 (24*
I am stuck here. I am confused about the heights given.
h1 is the height of the triangle, and the problem says that is 9 yd but from base to peak.
If i take the whole 9 yd= or 27 ft I will be taking part of the rectangular prism too. I don't think that is ok, or is it?.
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Would the height of the triangle be the half of the entire height , i mean half of 9 yd or 27 feet. Is that possible?.Yes, this is the missing dimension, but it's possible to calculate it. Look at the drawing of the whole barn and see which dimensions can help you.
and the other height 6 1/2 =6.5 is the height for the rectangular prism, not for the triangular prism or roof in this cae.
Last edited:
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
You wrote above: "If i take the whole 9 yd= or 27 ft I will be taking part of the rectangular prism too. I don't think that is ok, or is it?"Would the height of the triangle be the half of the entire height , i mean half of 9 yd or 27 feet. Is that possible?.
You are correct - this is not the way to go, because you are including the rectangular prism. So, if you want to exclude the rectangular prism from the 27 ft total, what should you do?
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Just find the feet from those 27 that represents the height of the triangular prism.You wrote above: "If i take the whole 9 yd= or 27 ft I will be taking part of the rectangular prism too. I don't think that is ok, or is it?"
You are correct - this is not the way to go, because you are including the rectangular prism. So, if you want to exclude the rectangular prism from the 27 ft total, what should you do?
Or take away the 6 1/2 ft (the height of the rectangular prism) from 27 ft.
Yes, this is the way to go. I feel i am right.
61/2 =6.5 ft
so , 27 ft -6.5 ft =20.5ft
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
Ok.20.5 is the height of the triangle or h1.
To avoid mistakes don't write 6 1/2 as 61/2 - sooner or later you'll think it's 61 divided by 2.
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Thanks, moving on to the other height, h220.5 is the height of the triangle or h1.
h2 is the height of our prism. to find the height of the prism I need to find the distance between the two bases. Let me think a little bit here.
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
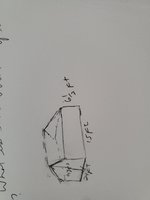
You need the distance between triangular bases. Please post a drawing with all dimensions marked so we know what you are referring to.Isn't the distance between the two bases of the rectangular prism the same as the height, 6 1/2?.
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
The problem says that the rectangular prism is 6 1/2 tall on both sides: for my untrained eye that looks as if the height between both bases is 6 1/2 or, the distance, in case we wanna change height for distance. For me distance and height are both the same thing here.You need the distance between triangular bases. Please post a drawing with all dimensions marked so we know what you are referring to.
Attachments
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
If you look at the shape as a barn, 6 1/2 will be the height of the 2 barn walls that are 15ft long. Makes sense? I don't see how it's relevant at this point.The problem says that the rectangular prism is 6 1/2 tall on both sides: for my untrained eye that looks as if the height between both bases is 6 1/2 or, the distance, in case we wanna change height for distance. For me distance and height are both the same thing here.
The height of the triangular prism is the distance between the 2 triangular bases. Which is what?
eddy2017
Elite Member
- Joined
- Oct 27, 2017
- Messages
- 2,525
Can I say that the distance between the two triangular bases is 15 ft ?.If you look at the shape as a barn, 6 1/2 will be the height of the 2 barn walls that are 15ft long. Makes sense? I don't see how it's relevant at this point.
The height of the triangular prism is the distance between the 2 triangular bases. Which is what?
lev888
Elite Member
- Joined
- Jan 16, 2018
- Messages
- 2,995
It is 15ft. But I don't quite understand your question. Are you asking for permission? Or guessing? There is no guessing in math. You concluded that it's 15ft - fine, don't be afraid to say it.Can I say that the distance between the two triangular bases is 15 ft ?.