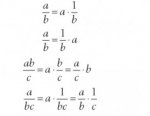Hi there,
I am currently working through Algebra Demystified and have come across some issues understanding the following:
At times, especially in calculus, students need to separate a variable from the rest of the fraction. This involves writing the fraction as a product of two fractions, or of one fraction and a whole number, or of one fraction and a variable.
Specifically, the book provides the following rules
. . . . .\(\displaystyle \large{ \dfrac{a}{b}\, =\, a\, \cdot\, \dfrac{1}{b} }\)
. . . . .\(\displaystyle \large{\dfrac{a}{b}\, =\, \dfrac{1}{b}\, \cdot\, a}\)
. . . . .\(\displaystyle \large{\dfrac{ab}{c}\, =\, a\, \cdot\, \dfrac{b}{c}\, =\, \dfrac{a}{c}\, \cdot\, b}\)
. . . . .\(\displaystyle \large{\dfrac{a}{bc}\, =\, a\, \cdot\, \dfrac{1}{bc}\, =\, \dfrac{a}{b}\, \cdot\, \dfrac{1}{c}}\)
I am really struggling to understand these - I can follow it from memory but I'd really like to be able to grasp the theory of this topic more. On a basic level, this makes sense to me:
. . . . .\(\displaystyle \large{\dfrac{x}{3}\, =\, \dfrac{1\, \cdot\, x}{3\, \cdot\, 1}\, =\, \dfrac{1}{3}\, \cdot\, \dfrac{x}{1}\, =\, \dfrac{1}{3}\,x}\)
. . . . .\(\displaystyle \large{\dfrac{a}{b}\, =\, \dfrac{1}{b}\, \cdot\, a}\)
But I am struggling with more complex fractions. Are there any good guides online which can explain in more detail how this works?
Thanks in advance.
I am currently working through Algebra Demystified and have come across some issues understanding the following:
At times, especially in calculus, students need to separate a variable from the rest of the fraction. This involves writing the fraction as a product of two fractions, or of one fraction and a whole number, or of one fraction and a variable.
Specifically, the book provides the following rules
. . . . .\(\displaystyle \large{ \dfrac{a}{b}\, =\, a\, \cdot\, \dfrac{1}{b} }\)
. . . . .\(\displaystyle \large{\dfrac{a}{b}\, =\, \dfrac{1}{b}\, \cdot\, a}\)
. . . . .\(\displaystyle \large{\dfrac{ab}{c}\, =\, a\, \cdot\, \dfrac{b}{c}\, =\, \dfrac{a}{c}\, \cdot\, b}\)
. . . . .\(\displaystyle \large{\dfrac{a}{bc}\, =\, a\, \cdot\, \dfrac{1}{bc}\, =\, \dfrac{a}{b}\, \cdot\, \dfrac{1}{c}}\)
I am really struggling to understand these - I can follow it from memory but I'd really like to be able to grasp the theory of this topic more. On a basic level, this makes sense to me:
. . . . .\(\displaystyle \large{\dfrac{x}{3}\, =\, \dfrac{1\, \cdot\, x}{3\, \cdot\, 1}\, =\, \dfrac{1}{3}\, \cdot\, \dfrac{x}{1}\, =\, \dfrac{1}{3}\,x}\)
. . . . .\(\displaystyle \large{\dfrac{a}{b}\, =\, \dfrac{1}{b}\, \cdot\, a}\)
But I am struggling with more complex fractions. Are there any good guides online which can explain in more detail how this works?
Thanks in advance.
Attachments
Last edited by a moderator: