whats the solution to these inequalities??
- Thread starter vlh
- Start date
D
Deleted member 4993
Guest
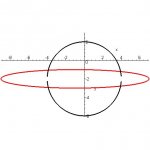
vlh said:x^2+(y+2)^2<16
(x+1)^2/64+(y+2)^2>1
Please show us your work, indicating exactly where you are stuck - so that we may know where to begin to help you.
BigGlenntheHeavy
Senior Member
- Joined
- Mar 8, 2009
- Messages
- 1,577
Hello, vlh!
Here's a start . . .
Multiply [2] by -1:Add [1]:And we have:which simplifies: −64(x+1)2−(y+2)2)x2+(y+2)2x2−64(x+1)263x2−2x−961<<<<−116150
This is an up-opening parabola. It is negative between its x-intercepts.
Quadratic Formula x=1262±242176≈{3.922-3.890}
Therefore: −3.890<x<3.922
. . which seems to agree with BigGlenn's graph
.
Here's a start . . .
\(\displaystyle \begin{array}{cccc}x^2+(y+2)^2 &<& 16 & [1] \\ \\[-3mm]\dfrac{(x+1)^2}{64}+(y+2)^2 &>& 1 & [2] \end{array}\)
Multiply [2] by -1:Add [1]:And we have:which simplifies: −64(x+1)2−(y+2)2)x2+(y+2)2x2−64(x+1)263x2−2x−961<<<<−116150
This is an up-opening parabola. It is negative between its x-intercepts.
Quadratic Formula x=1262±242176≈{3.922-3.890}
Therefore: −3.890<x<3.922
. . which seems to agree with BigGlenn's graph
.