volume rotating about a line

[mculve1]

Find the volume of the solid obtained by rotating the regioin enclosed by the graphs of y = e^ -x , y = 1- e ^-x , and
x = 0 about y = 4.


Help?

 

[Deleted member 4993]

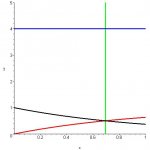
Find the volume of the solid obtained by rotating the regioin enclosed by the graphs of y = e^ -x , y = 1- e ^-x , and
x = 0 about y = 4.


Help?

First sketch the functions - find the points of intersections - define the limits.

Follow the method shown to you in:

viewtopic.php?f=3&t=39664

Please show us your work, indicating exactly where you are stuck - so that we may know where to begin to help you.

 

[mculve1]

intersects at .69314718

 

[mculve1]

excuse me that was very rude, im not trying to use you I have no idea how to do it or i wouldnt be on a calculus message board website..... wow.. that was very very rude for people that need help.


i dont even know what to say.

 

[BigGlenntheHeavy]

\(\displaystyle Washer:\)

\(\displaystyle \pi\int_{0}^{ln(2)}[(-3-e^{-x})^2-(e^{-x}-4)^2]dx \ = \ 6.748045943\)

\(\displaystyle Shell:\)

\(\displaystyle 2\pi\int_{0}^{.5}[(4-y)(-ln(1-y)]dy \ + \ 2\pi\int_{.5}^{1}[(4-y)(-ln(y))]dy \ = \ 6.748045943\)

\(\displaystyle See \ graph.\)

[attachment=0:tdqbsyvf]ghi.jpg[/attachment:tdqbsyvf]