Volume of a solid
- Thread starter JJ007
- Start date
Washer method?
Bottom function: fB(x)=0
Top function: \(\displaystyle f_T(x) = 2\sqrt{x}}\)
Distance to axis: r(x)=x
Use the following formula:
2π∫xaxbr(x)[(fT−fB)∘(x)]dx
In this case, we have
\(\displaystyle 2 \pi \int_{x_a = 0}^{x_b = 4} \left r(x)[(f_T-f_B)\circ (x) \right ]dx = 2 \pi \int_{0}^{4}x \left[ 2\sqrt{x}-0\right] dx\)
=4π∫04x3/2dx
Bottom function: fB(x)=0
Top function: \(\displaystyle f_T(x) = 2\sqrt{x}}\)
Distance to axis: r(x)=x
Use the following formula:
2π∫xaxbr(x)[(fT−fB)∘(x)]dx
In this case, we have
\(\displaystyle 2 \pi \int_{x_a = 0}^{x_b = 4} \left r(x)[(f_T-f_B)\circ (x) \right ]dx = 2 \pi \int_{0}^{4}x \left[ 2\sqrt{x}-0\right] dx\)
=4π∫04x3/2dx
BigGlenntheHeavy
Senior Member
- Joined
- Mar 8, 2009
- Messages
- 1,577
Shell:
2π∫04x(2x)dx = 5256π
Disc:
π∫04[42−(y2/4)2]dy = 5256π
2π∫04x(2x)dx = 5256π
Disc:
π∫04[42−(y2/4)2]dy = 5256π
Ok, I understand the shell method. But I'm little off on the disk part. Here's a similar example I tried with disk:
revolving region bounded by graphs of y=x3,y=1, and x=2 about the y-axis.
1=x3
x=1
v=π∫[R(x)]2dx
v=π∫12[x3]2dx=7127π
or
v=π∫12[x3−1]2dx
v=π∫12[x6−2x3+1]dx=14163π?
The real answer is supposed to be 120/7 pi
Thanks guys.
revolving region bounded by graphs of y=x3,y=1, and x=2 about the y-axis.
1=x3
x=1
v=π∫[R(x)]2dx
v=π∫12[x3]2dx=7127π
or
v=π∫12[x3−1]2dx
v=π∫12[x6−2x3+1]dx=14163π?
The real answer is supposed to be 120/7 pi
Thanks guys.
JJ007 said:Ok, I understand the shell method. But I'm little off on the disk part. Here's a similar example I tried with disk:
revolving region bounded by graphs of y=x3,y=1, and x=2 about the y-axis.
1=x3
x=1
v=π∫[R(x)]2dx
v=π∫12[x3]2dx=7127π
or
v=π∫12[x3−1]2dx
v=π∫12[x6−2x3+1]dx=14163π?
The real answer is supposed to be 120/7 pi
Thanks guys.
Neither.
R2(x)−r2(x)=(x3)2−12.
V=π∫12(x6−1)dx
BigGlenntheHeavy
Senior Member
- Joined
- Mar 8, 2009
- Messages
- 1,577
Note: This is revolving about the Y−AXIS.
Shell:
2π∫12(x3−1)xdx = 547π
Washer:
π∫18(4−y2/3)dy = 547π
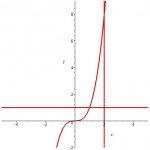
See graph below:
[attachment=0:jwq63sta]mno.jpg[/attachment:jwq63sta]
This is revolving about the X−AXIS
Shell:
2π∫18y(2−y1/3)dy = 7120π
Washer:
π∫12(x6−1)dx = 7120π
Shell:
2π∫12(x3−1)xdx = 547π
Washer:
π∫18(4−y2/3)dy = 547π
See graph below:
[attachment=0:jwq63sta]mno.jpg[/attachment:jwq63sta]
This is revolving about the X−AXIS
Shell:
2π∫18y(2−y1/3)dy = 7120π
Washer:
π∫12(x6−1)dx = 7120π