Volume of a solid: CROSS SSCTIONS

[Brain0991]

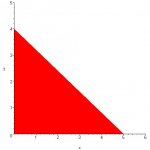
A solid has a base bounded by the line 4x + 5y = 20, x = 0, and y = 0. Cross sections perpendicular to the x-axis are semicircles. The Diameter of each semicircle is the length of the segment connecting a point on the x-axis to the line 4x + 5y = 20. Find the volume of the resulting solid using the method of cross section.

Then it shows a picture of the graph of 4x + 5y = 20 with two lines at x = 7.5 and x = -7.5, and a spring going for the x-axis to the graph at x = 2.5.

Volume = ?A(x)dx from a to b

I honestly do not even know what this means, my book is extremely vague in explaining the Cross section technique, and the only example I have is a triangular cross section.

I think I have to start by using the Disk Method.
??(4-(4x/5))[sup:1f7xjlqk]2[/sup:1f7xjlqk] dx
??16 - 32x/5 - (16x[sup:1f7xjlqk]2[/sup:1f7xjlqk])/25 dx
?(16x - (8x[sup:1f7xjlqk]2[/sup:1f7xjlqk])/5 - (16x[sup:1f7xjlqk]3[/sup:1f7xjlqk])/75) from 0 to 5
= 41.89

But from here, I do not know where to go?

 

[BigGlenntheHeavy]

\(\displaystyle A_{semi circle} \ = \ \frac{\pi r^2}{2}\)

\(\displaystyle Hence, \ V \ = \ \frac{\pi}{2}\int_{a}^{b}r^2 \ dx\)

\(\displaystyle Can \ you \ take \ it \ from \ here?\)

\(\displaystyle Here \ is \ the \ base \ of \ the \ graph.\)

[attachment=0:3cs1jxe4]fff.jpg[/attachment:3cs1jxe4]

 

[galactus]

I am thinking along the same lines as Glenn. But, what is the 7.5 for?. And what is the 'spring'?.