Volume of a solid by rotating.

[Chris*]

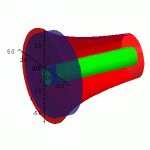
Here's the problem I have.
Consider the solid obtained by rotating the region bounded by the given curves about the line y = -1
Given curves: \(\displaystyle y=\frac{4}{x}, y=0, x=1, x=3\)

Find the volume V of this solid.

I sketched the the graph of the function and found the radii where the "inner" radius = 1 and the "outer" radius = \(\displaystyle 1+\frac{4}{x}\)

And from there I got:
\(\displaystyle V=\int_{1}^{3}\pi((1+\frac{4}{x})^2-1^2))dx\)

However, when I try to integrate it, I get an undefined number.

\(\displaystyle \int_{1}^{3}\pi((1+\frac{4}{x})^2-1^2))dx\)

\(\displaystyle =\pi \int_{1}^{3}((1+\frac{8}{x}+\frac{16}{x^2})-1)dx\)

\(\displaystyle =\pi \int_{1}^{3}(\frac{8}{x}+\frac{16}{x^2})dx\)

\(\displaystyle =\pi (\frac{8x^{(-1+1)}}{-1+1}}+\frac{16x^{(-2+1)}}{-2+1})\mid _{1}^{3}\)

I thought I knew how to solve the problem, but I'm not sure which part I messed up, so any help would be appreciated.

 

[galactus]

You have the correct set up. I think you are just making some integration errors.

I am sorry, but I do not follow your last line. What are those values in the exponents for?

It simplifies to

\(\displaystyle {\pi}\int_{1}^{3}\left[\frac{8}{x}+\frac{16}{x^{2}}\right]dx=\frac{8\pi(xln(x)-2)}{x}\)

\(\displaystyle \frac{8{\pi}(3ln(3)-2)}{3}-\frac{8{\pi}(ln(1)-2)}{1}=\frac{8{\pi}(3ln(3)+4)}{3}\approx 61.12\)

You are correct. I think the problem is when you sub in your integration limits.

 

[Chris*]

Ah I see... In my calculus class, we haven't been taught any integration that involves logs. I took the anti-derivatives by changing \(\displaystyle \frac {8}{x}\) to \(\displaystyle 8{x^{-1}\) and \(\displaystyle \frac{16}{x^2}\) to \(\displaystyle 16x^{-2}\) and then adding 1 to the power and putting the sum of the two exponents in the denominator.

but it seemed impossible to integrate \(\displaystyle \frac {8}{x}\) this way.

I may be in trouble if my instructor starts putting these things on the exam all of a sudden... :|

 

[galactus]

The derivative of ln(x) is 1/x. Therefore, the antiderivative of 1/x is ln(x). Keep these in mind. They are to be remembered. They will pop up again and again. Trust me.