Undefinable? or Convergent...

[klooless]

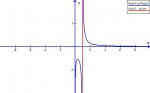
This is my question;

?dx/xln(x) dx from [1,2]

My answer is: [ln(ln2) - ln(0)]

Here I am wondering if the integral is convergent since ln(0) is undefinable and is therefore considered as "0" making the integral ln(ln2),
Or
divergent since as x approaches 0 the integral is negative infinity?

I am leaning towards divergent, but I don't know if I have the right reasoning for this.

Thanks!

 

[pka]

This
I am leaning towards divergent, but I don't know if I have the right reasoning for this.

Note that \(\displaystyle \frac{1}{x\ln(x)}\) is not defined for \(\displaystyle x=1\).
Therefore it is an improper integral.
You are correct.

 

[BigGlenntheHeavy]

The improper integral diverges as limit ln|0| approaches -infinity.

 

[Deleted member 4993]

is the problem

\(\displaystyle \int^2_1\frac{dx}{x}ln(x)\)

or

\(\displaystyle \int^2_1\frac{dx}{x \, ln(x)}\)

 

[klooless]

the second one...

 

[galactus]

Here is a graph of \(\displaystyle \frac{1}{xln(x)}\). See why it diverges at 1?. It has a vertical asymptote at x=1.

So, if the limits of integration are 1 to 2, then it diverges.

\(\displaystyle \int_{L}^{2}\frac{1}{xln(x)}dx=ln(ln(x))|_{L}^{2}=ln(ln(2))-ln(ln(L))\)

\(\displaystyle \lim_{L\to 1^{+}}ln(ln(L))=-\infty\)

\(\displaystyle ln(ln(2))-(-\infty)={\infty}\)