triple integral for volume of region

[jwpaine]

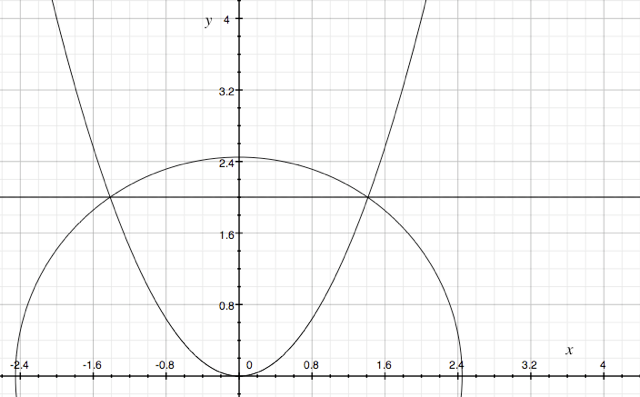
Set up the triple integral for the volume of the region bounded below by the paraboloid: z = x^2 + y^2 and above by the sphere x^2 + y^2 + z^2 = 6

This was my attempt:

The two equations bind a spherical slice of the r = \(\displaystyle \sqrt{6}\) larger sphere, defined by 360 degrees of base rotation, \(\displaystyle \phi\) between 0 and 90 degrees, giving region:

\(\displaystyle 0 \leq \theta \leq 2 \pi\),
\(\displaystyle 0 \leq \phi \leq \frac{\pi}{2}\),
\(\displaystyle sec\theta \leq r \leq \sqrt{6} \cdot cos\theta\)

Thus the volume of that region would be:

\(\displaystyle \int_0^{2\pi} \int_0^{\frac{\pi}{2}} \int_{sec\theta}^{ \sqrt{6}sec \theta} p^2 sin \theta dP d\phi d\theta\)

Does this look right? If not, what did I do wrong or where should I go from here?

Thanks,
John

 

[galactus]

Hey JW.

I think yours evaluates to infinity.

Note that for the sphere \(\displaystyle {\rho}=\sqrt{6}\)

From \(\displaystyle z=x^{2}+y^{2}\):

\(\displaystyle {\rho}cos{\phi}={\rho}^{2}sin^{2}{\phi}cos^{2}{\theta}+{\rho}^{2}sin^{2}{\phi}sin^{2}{\theta}\)

It whittles down to \(\displaystyle {\rho}=cot{\phi}csc{\phi}\)

Now, can you get it. Do you have to use spherical?. It may be easier in cylindrical.

 

[jwpaine]

galactus,


Here is the image of the region bounded by the intersection of both functions:

Which leaves a volume region that is sliced from the top of the sphere by the paraboloid.

Is this what needs to be integrated, or is it the region as shown below (then rotated 360 degrees?) I'm a bit confused here.

Which in that case, I would say, with cylindrical coords: 0 <= t <= 2pi, 0 <= r <= sqrt(2), 0 <= z <= sqrt(6)

Right?
Thanks for your time,
John

 

[Deleted member 4993]

I would divide the regions into two parts ( spherecal cap at the top and paraboloid at the bottom) and simply use disk method (dV = ? * r[sup:11h1g4qe]2[/sup:11h1g4qe] dz) and integrate.

 

[galactus]

Yes, solids of revolution is always a good choice, but I would assume this is calc III and triple integrals are the issue.

Here it is in rectangular:

\(\displaystyle \int_{-\sqrt{2}}^{\sqrt{2}}\int_{-\sqrt{2-x^{2}}}^{\sqrt{2-x^{2}}}\int_{x^{2}+y^{2}}^{\sqrt{6-x^{2}-y^{2}}}dzdydx\)

If you use SK's suggestion of a solid of revolution you should get the same result.

\(\displaystyle 2{\pi}\int_{0}^{\sqrt{2}}x(\sqrt{6-x^{2}}-x^{2})dx\)


Then, try it in polar and spherical to see if you get the same.

polar:

\(\displaystyle \int_{0}^{2\pi}\int_{0}^{\sqrt{2}}\int_{r^{2}}^{\sqrt{6-r^{2}}}rdzdrd{\theta}\)

I don't think this one is easy to express in spherical. Because an arrow shot from the origin could leave the region at a point on either the sphere or the cylinder.