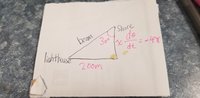
A lighthouse is situated 200m from a straight shoreline. The light rotates clockwise at 2 revolutions per minute. At what speed is the beam of light moving along the shore when the angle between the beam and the nearest point on the shore is 30 degree?
Would you solve with 30 degree or 60 degree and why?
Would you solve with 30 degree or 60 degree and why?