The Washer Method: Q1, y=6, y=sqrt[6x], y-axis, about y=6

[Shutterbug424]

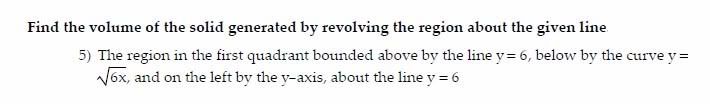
Using the washer method (V= pi * int from a to b (R(x)^2 - r(x)^2) dx

I determined R(x) to be (6- sqrt(6x)) and r(x) to be 0

After working out the problem, I got 36 pi.

I have no way of checking my answers, so I was hoping that some nice person could verify it for me.

If I went wrong, it was probably in taking the integral of 2(6x)^(1/2)...so if someone could at least tell me what the integral of that is that would be great!

 

[galactus]

Re: The Washer Method

Looks good. Excellent work. We can even do it with shells to see if we get the same.

Shells:

\(\displaystyle 2{\pi}\int_{0}^{6}[(6-y)(\frac{x^{2}}{6})]dy=36{\pi}\)


Washers:

\(\displaystyle {\pi}\int_{0}^{6}(\sqrt{6x}-6)^{2}dx=36{\pi}\)

 

[Shutterbug424]

Re: The Washer Method

Thanks a bunch!