BigGlenntheHeavy
Senior Member
- Joined
- Mar 8, 2009
- Messages
- 1,577
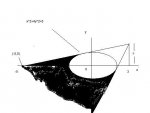
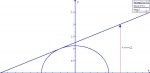
The figure shows a lamp located three units to the right of the y−axis and a
shadow created by the elliptical region x2+4y2 = 5. If the point (−5,0) is on
the edge of the shadow, how far above the x−axis is the lamp located?
[attachment=0:3ibb9tb8]AAA.JPG[/attachment:3ibb9tb8]
shadow created by the elliptical region x2+4y2 = 5. If the point (−5,0) is on
the edge of the shadow, how far above the x−axis is the lamp located?
[attachment=0:3ibb9tb8]AAA.JPG[/attachment:3ibb9tb8]