Tangent to the Quadratic

B

Bobby Jones

New member
Joined
Jul 8, 2011
Messages
41
Morning people, what is meant be the wording, "is a tangent to the quadratic equation"

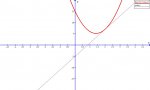
The quetion asks: show that the line y=6x-5 is a tangent to the quadratic equation f(x) = 5x^2-14x+15 and find the co-ordinates of the point of contact.

Is this correct to say

y=5x^2-14x+15
y=6x-5

Therefore, 6x-5 = 5x^2-14x+15
5x^2-20x-20=0

(5x-10)(x-2)

x=2

Therefore, y=6(2)-5
y=7

Co-ordinates of where they meet is (2,7)

I just dont know whats meant by the wording of tangent in this case?
 
What does tangent mean in some other context? Think of a circle with a line through it. If there are two points of intersection, it is SECANT with the circle. Pull the line around a bit until there is only one point of intersetion. Now it is TANGENT with the circle.
 
Since you solved it, here is a graph of the tangent line to the parabola at (2,7).

Do you have a graphing calculator?. If so, graph your functions and see what it looks like.

If not, go here and download the free graphing utility:

www.padowan.dk
 

Attachments

  • tangent.jpg
    tangent.jpg
    34.6 KB · Views: 92


Bobby Jones said:
what is meant [by] the wording, "is a tangent to the quadratic equation"

This is sloppily worded; equations do not have tangent lines.



"show that the line y=6x-5 is a tangent to the quadratic equation f(x) = 5x^2-14x+15"

Again, what they are trying to say is:

"show that the line y = 6x - 5 is tangent to the graph of the quadratic function f(x) = 5x^2 - 14x + 15"



Co-ordinates of where they meet is (2, 7)

This is correct for that part of the exercise, but you have not yet shown that the given line is tangent to the graph.

Demonstrate that the slope of the line is equal to the slope of the graph of f(x) at the point (2, 7).

This confirms that the given line is a tangent line.

Cheers 8-)
Click to expand...

PS: Here's a web-based graphing utility (i.e., no software download or installation required).

 
How do I do that? how do i demonstrate that the line is tangent to the curve? I've found the co-ordinates, but should I draw a picture to show that they are tangent or is there a mathematical way to show this?
 
There are only three possible outcomes.

1) No intersection point.
2) One Intersection Point (or the same one twice) ==> Tangent
3) Two intersection points (distinct) ==> Secant
 
Very cautiously, I dare to disagree with tkhunny.

To show that a line is the tangent line to a smooth curve at point a, you must show that the line intersects the curve at a. The line must have a number of other properties, such as not intersecting the curve again close to a. Now if the line intersects the curve ONLY at a, it obviously does not intersect the curve at points close to a. Differential calculus is mostly about what happens over a small domain around a point on a curve. It is LOCAL, not GLOBAL, but sometimes the global solution and local solution are the same.

EDIT MADE: I originally tried to give an exhaustive list of the properties required for a line to be a tangent to a curve at a given point. I failed. I have modifed my post to point out that there are a number of required properties and that two of them are directly relevant to tkhunny's explanation. See: I knew I should not have disagreed with him.
 
Ya gonna have to chuck me some hints, should I differentiate the 5x^2-14x+15 to make 10x-14, but what then! how does that prove that the line is tangent to the curve?
 
How many solutions did you get when you set the line equation and the quadratic equal to one another?. One.

If the line intersects the curve in more than one place, then you have two distinct solutions.
 
Bobby Jones said:
Ya gonna have to chuck me some hints, should I differentiate the 5x^2-14x+15 to make 10x-14, but what then! how does that prove that the line is tangent to the curve?
Click to expand...
You didn't read what I wrote, did you?
 
Bobby Jones said:
Ya gonna have to chuck me some hints, should I differentiate the 5x^2-14x+15 to make 10x-14, but what then! how does that prove that the line is tangent to the curve?
Click to expand...

The "value" of the derivative of a function (one variable) at a point - is the "slope" of the tangent line (to the graph of the function) at that point.
 
OK I am here to apologize to tkhunny, and I have edited my original post.
 
You must log in or register to reply here.
Top