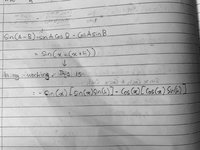You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Stuck on L'Hopital Trigono
- Thread starter Jacob
- Start date
Jacob
New member
- Joined
- Jan 27, 2019
- Messages
- 41
After hours of research, I finally know where I stuck.
Now I want to know what happened to cos(x) cos(x) sin(h). In Yt or other website I see Sin(A-B) method is commonly used in but no idea what happening to cos(x) cos(x) sin(h).
Also, I know there’s few mistakes in my previous work. Can someone explain
Now I want to know what happened to cos(x) cos(x) sin(h). In Yt or other website I see Sin(A-B) method is commonly used in but no idea what happening to cos(x) cos(x) sin(h).
Also, I know there’s few mistakes in my previous work. Can someone explain
Attachments
Dr.Peterson
Elite Member
- Joined
- Nov 12, 2017
- Messages
- 16,749
Can someone please show and explain to me how I can get the final answer as -1/sin²x. Really been trying to solve this for 2days now and my lecturer isn’t helpful?.
Also, is my working correct?
Here's an error:

Here's another:
And there are more. At one point you canceled sin x from only one term, which is illegal.
I'm not sure what you're saying here. And I don't see how the work you show would fit it.After hours of research, I finally know where I stuck.
Now I want to know what happened to cos(x) cos(x) sin(h). In Yt or other website I see Sin(A-B) method is commonly used in but no idea what happening to cos(x) cos(x) sin(h).
Fix the original version, and eventually you will be able to apply the limit of sin(x)/x.
Jacob
New member
- Joined
- Jan 27, 2019
- Messages
- 41
Yes my apologies, I was careless. I finally understood how to get the answer but not sure what is happening to the red part that I highlighted.Here's an error:
View attachment 25043
Here's another:
View attachment 25045
And there are more. At one point you canceled sin x from only one term, which is illegal.
I'm not sure what you're saying here. And I don't see how the work you show would fit it.
Fix the original version, and eventually you will be able to apply the limit of sin(x)/x.

Dr.Peterson
Elite Member
- Joined
- Nov 12, 2017
- Messages
- 16,749
Yes my apologies, I was careless. I finally understood how to get the answer but not sure what is happening to the red part that I highlighted.
View attachment 25047
The boxed part is copied directly from the line above. What confuses you about that? But what follows is not shown in enough detail; are you saying you copied this work from someone else, and are trying to understand it?
The next thing to do after that line is to factor out sin(h). The remaining factor should look Pythagorean to you ... which will lead to the next line.
Jacob
New member
- Joined
- Jan 27, 2019
- Messages
- 41
Above the purple line Its my own working but below it I referred to Websites & Youtube.The boxed part is copied directly from the line above. What confuses you about that? But what follows is not shown in enough detail; are you saying you copied this work from someone else, and are trying to understand it?
The next thing to do after that line is to factor out sin(h). The remaining factor should look Pythagorean to you ... which will lead to the next line.
About the box part. What happens to it after? Is it that part gives me 1/sin(x)•sin(x+h)? Because in Websites and Yt there’s no explanation or they skip some steps perhaps that’s why I’m still confused about the box part. Also, I don't know from where they got 1/sin(x)•sin(x+h)

Last edited:
Dr.Peterson
Elite Member
- Joined
- Nov 12, 2017
- Messages
- 16,749
Do you not see that, as I suggested, both terms on that line contain sin(h), so you can factor that out? Please do that, and the rest should become clear!Above the purple line Its my own working but below it I referred to Websites & Youtube.
About the box part. What happens to it after? Is it that part gives me 1/sin(x)•sin(x+h)? Because in Websites and Yt there’s no explanation or they skip some steps perhaps that’s why I’m still confused about the box part. Also, I don't know from where they got 1/sin(x)•sin(x+h)
Your arrow skips a line; the second term in the one line can't become the second factor in the second line after it, can it? Just take one step at a time, and I promise you, it will become clear. You'll get the first line under the purple, and then that line can be split up as a product, by just pulling out the sin(h)/h as a factor (because you should know that limit).
Jacob, the numerator from your third line down along your left margin is
sin(x)[cos(x + h)] - cos(x)[sin(x + h)]
Looking at math tutorials on the Internet, let angle A = x and let angle B = (x + h).
Then this is of the form sin(A - B) = sin[x - (x + h)] = sin(-h) = -sin(h).
This method in the tutorials, as you can see, does not use L'Hopital's Rule.
(I see that your way does not use that rule either, so I don't know why you have it in the subject line.)
sin(x)[cos(x + h)] - cos(x)[sin(x + h)]
Looking at math tutorials on the Internet, let angle A = x and let angle B = (x + h).
Then this is of the form sin(A - B) = sin[x - (x + h)] = sin(-h) = -sin(h).
This method in the tutorials, as you can see, does not use L'Hopital's Rule.
(I see that your way does not use that rule either, so I don't know why you have it in the subject line.)
Last edited:
Jacob
New member
- Joined
- Jan 27, 2019
- Messages
- 41
Im sorry been looking for the common term since till I confused myself with a lot things since I can't see it clearly.Do you not see that, as I suggested, both terms on that line contain sin(h), so you can factor that out? Please do that, and the rest should become clear!
Your arrow skips a line; the second term in the one line can't become the second factor in the second line after it, can it? Just take one step at a time, and I promise you, it will become clear. You'll get the first line under the purple, and then that line can be split up as a product, by just pulling out the sin(h)/h as a factor (because you should know that limit).
Jacob
New member
- Joined
- Jan 27, 2019
- Messages
- 41
My lecturer didn't explain whether it"s L'Hopital's rule or the First Principle of Derivative. All I know is that I got to solve the problem using f(x+h)-f(x)/h and sinh/h = 1 & Cosh-1/h =0.Jacob, the numerator from your third line down along your left margin is
sin(x)[cos(x + h)] - cos(x)[sin(x + h)]
Looking at math tutorials on the Internet, let angle A = x and let angle B = (x + h).
Then this is of the form sin(A - B) = sin[x - (x + h)] = sin(-h) = -sin(h).
This method in the tutorials, as you can see, does not use L'Hopital's Rule.
(I see that your way does not use that rule either, so I don't know why you have it in the subject line.)
His explanation pretty bad so I had to look it up on the internet. He wants me to prove that cotx = -csc^2x
Last edited:
Dr.Peterson
Elite Member
- Joined
- Nov 12, 2017
- Messages
- 16,749
You are finding the derivative from the definition, which is often called "first principles". And, no, you aren't proving that cotx = -csc^2x, but that the derivative of cotx is -csc^2x. That's an important distinction.My lecturer didn't explain whether it"s L'Hopital's rule or the First Principle of Derivative. All I know is that I got to solve the problem using f(x+h)-f(x)/h and sinh/h = 1 & Cosh-1/h =0.
His explanation pretty bad so I had to look it up on the internet. He wants me to prove that cotx = -csc^2x
Are you saying you can't see this common factor???Im sorry been looking for the common term since till I confused myself with a lot things since I can't see it clearly.
When you factor that out, you get \(-\sin(h)[\sin^2(x)+\cos^2(x)] = -\sin(h)\), because \(\sin^2(x)+\cos^2(x) =1\). That's what's on the next line.