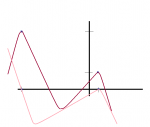
Sketch a graph of a function f(x) that is differentiable and that satisfies the following conditions.
f′(x)>0, when x<−5
f′(x)<0, when −5<x<1 and when x>1
f′(−5)=0 and f′(1)=0
f(−5)=6 and f(1)=2
Did I do this question correctly, if not, please fix my mistake!
Legend:
f′(x) is pink
f(x) is red
f′(x)>0, when x<−5
f′(x)<0, when −5<x<1 and when x>1
f′(−5)=0 and f′(1)=0
f(−5)=6 and f(1)=2
Did I do this question correctly, if not, please fix my mistake!
Legend:
f′(x) is pink
f(x) is red