simple algebra at the end of a calc problem

Hello, CalcGuy!

I see nothing wrong with your answer.


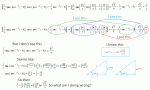
We have:  12⋅sin⁡[sec⁡−1(−9)]⋅cos⁡[sec⁡−1(−9)]\displaystyle \text{We have: }\:\frac{1}{2}\cdot\sin\left[\sec^{-1}(-9)\right]\cdot\cos\left[\sec^{-1}(-9)\right]


Let:  θ = sec⁡−1(−9)⇒sec⁡θ = 9−1 = hypadj\displaystyle \text{Let: }\:\theta \,=\,\sec^{-1}(-9) \quad\Rightarrow\quad \sec\theta \:=\:\frac{9}{-1} \:=\:\frac{hyp}{adj}

So, θ is in a right triangle with:  adj=−1,  hyp=9\displaystyle \text{So, }\theta\text{ is in a right triangle with: }\:adj = -1,\;hyp = 9


\(\displaystyle \text{Assuming }\theta\text{ is in Quadrant 3, we have: }\:eek:pp \,=\,-\sqrt{80}\,=\,-4\sqrt{5}\)

Hence:  sin⁡θ = −459,    cos⁡θ = −19\displaystyle \text{Hence: }\:\sin\theta \:=\:-\frac{4\sqrt{5}}{9},\;\;\cos\theta \:=\:-\frac{1}{9}


Therefore:  12sin⁡θcos⁡θ  =  12(−459)(−19)  =  2581\displaystyle \text{Therefore: }\:\frac{1}{2}\sin\theta\cos\theta \;=\;\frac{1}{2}\left(-\frac{4\sqrt{5}}{9}\right)\left(-\frac{1}{9}\right) \;=\;\frac{2\sqrt{5}}{81}

 
Note: arcsec(−9) = arcsin(1/9)+π/2\displaystyle Note: \ arcsec(-9) \ = \ arcsin(1/9)+\pi/2

Also note: ∫−2−9x2−1x3dx = −∫−9−2x2−1x3dx\displaystyle Also \ note: \ \int_{-2}^{-9}\frac{\sqrt{x^{2}-1}}{x^{3}}dx \ = \ -\int_{-9}^{-2}\frac{\sqrt{x^{2}-1}}{x^{3}}dx

Is your Professor a trickster?\displaystyle Is \ your \ Professor \ a \ trickster?

Hence, final solution = (1/2)[t−sin(t)cos(t)]arcsin(1/9)+π/22π/3\displaystyle Hence, \ final \ solution \ = \ (1/2)[t-sin(t)cos(t)]_{arcsin(1/9)+\pi/2}^{2\pi/3}

= 12[2π3−(32)(−12)−[arcsin(1/9)+π2−(459)(−19)]]\displaystyle = \ \frac{1}{2}\bigg[\frac{2\pi}{3}-(\frac{\sqrt3}{2})(\frac{-1}{2})-[arcsin(1/9)+\frac{\pi}{2}-(\frac{4\sqrt5}{9})(\frac{-1}{9})]\bigg]

= 12[2π3+34−arcsin(1/9)−π2−4581] = about .367424\displaystyle = \ \frac{1}{2}\bigg[\frac{2\pi}{3}+\frac{\sqrt3}{4}-arcsin(1/9)-\frac{\pi}{2}-\frac{4\sqrt5}{81}\bigg] \ = \ about \ .367424
 
When I evaluate the given equation, I don't get equality.

0.3674 ? 0.2381
 
Note: For what it is worth dept., just my opinion.\displaystyle Note: \ For \ what \ it \ is \ worth \ dept., \ just \ my \ opinion.

When working with trig. functions, I always (if possible) try to break them down into sin, cos,\displaystyle When \ working \ with \ trig. \ functions, \ I \ always \ (if \ possible) \ try \ to \ break \ them \ down \ into \ sin, \ cos,

 or tan functions and also get rid of any negative signs, if possible; as this will make it\displaystyle \ or \ tan \ functions \ and \ also \ get \ rid \ of \ any \ negative \ signs, \ if \ possible; \ as \ this \ will \ make \ it

 easier, I have found to solve.\displaystyle \ easier, \ I \ have \ found \ to \ solve.

For example: sec(t) = −9, 1cos(t) = −9, cos(t) = −19\displaystyle For \ example: \ sec(t) \ = \ -9, \ \frac{1}{cos(t)} \ = \ -9, \ cos(t) \ = \ \frac{-1}{9}

sin(π2−t) = −19, π2−t = arcsin(−19)\displaystyle sin(\frac{\pi}{2}-t) \ = \ \frac{-1}{9}, \ \frac{\pi}{2}-t \ = \ arcsin(\frac{-1}{9})

t = π2−arcsin(−19) = π2+arcsin(19)\displaystyle t \ = \ \frac{\pi}{2}-arcsin(\frac{-1}{9}) \ = \ \frac{\pi}{2}+arcsin(\frac{1}{9})

Last one: Let k = −arcsin(−19), then −k = arcsin(−19)\displaystyle Last \ one: \ Let \ k \ = \ -arcsin(\frac{-1}{9}), \ then \ -k \ = \ arcsin(\frac{-1}{9})

Hence, sin(−k) = (−19), −sin(k) = (−19),   ⟹   sin(k) = 19, k = arcsin(1/9)\displaystyle Hence, \ sin(-k) \ = \ (\frac{-1}{9}), \ -sin(k) \ = \ (\frac{-1}{9}), \ \implies \ sin(k) \ = \ \frac{1}{9}, \ k \ = \ arcsin(1/9)

Therefore, −arcsin(−19) = k = arcsin(19)\displaystyle Therefore, \ -arcsin(\frac{-1}{9}) \ = \ k \ = \ arcsin(\frac{1}{9})

All this grunt work could have been avoided, by just punching in arccos(1/−9) into your\displaystyle All \ this \ grunt \ work \ could \ have \ been \ avoided, \ by \ just \ punching \ in \ arccos(1/-9) \ into \ your

 trusty TI−89 and you would have gotten  arcsin(1/9) + π/2.\displaystyle \ trusty \ TI-89 \ and \ you \ would \ have \ gotten \ \ arcsin(1/9) \ + \ \pi/2.

The TI−89 tells no lies, the TI−89 knows best.\displaystyle The \ TI-89 \ tells \ no \ lies, \ the \ TI-89 \ knows \ best.
 
You must log in or register to reply here.
Top