similar triangles/proportions

[dazed and confused]

really confused on this:

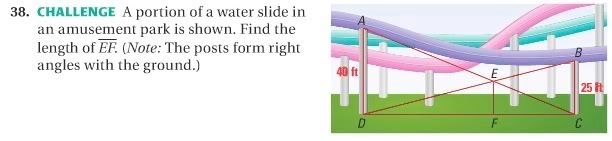
it doesn't make much sense to me. i feel like i need another side length anywhere. now i can figure out all the similarities between the triangles (ACD ~ ECF; BDC ~ EDF; EBC ~ EDA)... i just have no clue how the heck I am supposed to get this.

can anyone push me in the right direction?

 

[wjm11]

Try writing down some things you know are true due to the similar triangles you have identified:

EF/FC = 40/DC
Therefore, EF = 40(FC)/DC

EF/DF = 25/DC
Therefore, EF = 25(DF)/DC

Therefore, 40(FC)/DC = 25(DF)/DC
And 40(FC) = 25(DF)
So DF/FC = 40/25 = 8/5

This means that the ratio of DF/FC is constant, regardless of the distance between the support posts, AD and BC. Therefore, one can choose an arbitrary distance between them, such as “13” and let DF = 8 and FC = 5 (maintaining the DF/FC ratio).

You should be able to solve for EF now.

 

[dazed and confused]

i'm more confused now.

are you trying to say EF is 25? because it just can't be because that would make triangle BCD be a square.

 

[wjm11]

EF is not 25. I repeat:

This means that the ratio of DF/FC is constant, regardless of the distance between the support posts, AD and BC. Therefore, one can choose an arbitrary distance between them, such as “13” and let DF = 8 and FC = 5 (maintaining the DF/FC ratio).

Solve for EF using ratios based on similar triangles with the DF and FC values I suggested. Please show me your work if you have any additional questions.