Cambridge101
New member
- Joined
- Dec 16, 2021
- Messages
- 49
Ok so just for background, I am completely a self taught math lover, ( I am going to Cambridge university to study Economics in September), but until then I have been teaching myself higher level maths while going over old stuff I never was taught as a kid and wanted to fully understand.
I had one particularly random shower thought to do with the speed distance and time.
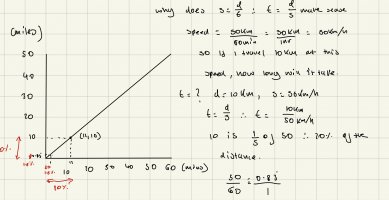
Ok so, say I am travelling 50km/h. And I graph this (on a distance time graph) to show that in 60 mins I travel 50km. Speed is constant and the gradient is 50/1 or 50/60 for this graph = 5/6. So for every 6 mins in time, we travel 5km. What interested me is that 5 is 10% of 50 and 6 is 10% of 60. They're equally as far along their axes as each-other.
Now people are taught simply s = d/t therefore, t = d/s (juts learn it). But I want to know intuitively why this rearrangement makes sense. But because I learn all my maths alone I have to try and make connections myself.
In my case say I travel 10km at a speed of 50km/h and I want to find the time is took.
So using the formula t = 10km / 50km/h
They way I think about this is this, if were saying 10/50 this is 10 out of 50. Thus, 10 km is 1/5th of 50km as a distance, 20%. So you can see as shown on my graph. A distance of 10km is 20% of the total distance traveled in the time of 1 hr. So because the answer to is 1/5 or 0.2hr because if it is 20% of the distance it must take 20% of the time? Because to me it doesn't seem to make intuitive sense for some reason - I just cant see the connect as to why. The answer is 12 mins when you plug it into the formula and 12 is also 20% of 60 mins. So it is like if we go 20% up the y-axis, we go 20% up the x-axis. Likewise, if we simply the ratio to 0.83 recurring / 1 I find that these are also both worth 1.6 recurring % of their axis lengths. Why is this?
Thank you guys.
I had one particularly random shower thought to do with the speed distance and time.
Ok so, say I am travelling 50km/h. And I graph this (on a distance time graph) to show that in 60 mins I travel 50km. Speed is constant and the gradient is 50/1 or 50/60 for this graph = 5/6. So for every 6 mins in time, we travel 5km. What interested me is that 5 is 10% of 50 and 6 is 10% of 60. They're equally as far along their axes as each-other.
Now people are taught simply s = d/t therefore, t = d/s (juts learn it). But I want to know intuitively why this rearrangement makes sense. But because I learn all my maths alone I have to try and make connections myself.
In my case say I travel 10km at a speed of 50km/h and I want to find the time is took.
So using the formula t = 10km / 50km/h
They way I think about this is this, if were saying 10/50 this is 10 out of 50. Thus, 10 km is 1/5th of 50km as a distance, 20%. So you can see as shown on my graph. A distance of 10km is 20% of the total distance traveled in the time of 1 hr. So because the answer to is 1/5 or 0.2hr because if it is 20% of the distance it must take 20% of the time? Because to me it doesn't seem to make intuitive sense for some reason - I just cant see the connect as to why. The answer is 12 mins when you plug it into the formula and 12 is also 20% of 60 mins. So it is like if we go 20% up the y-axis, we go 20% up the x-axis. Likewise, if we simply the ratio to 0.83 recurring / 1 I find that these are also both worth 1.6 recurring % of their axis lengths. Why is this?
Thank you guys.
Attachments
Last edited: