Quadratic real life application

[adicus22]

The assignment is below, followed by my creative atempt to answer the question. Math is definately not my strong point but I refuse to allow that to stop me from getting at least a B in this College Algebra class. I am shooting for an A but I will settle for a B. I like to think outside the box but have a feeling that my function does not exactly match up properly to what my assignment is asking for. The teacher used the equation for an object being thrown straight up into the air and said we are not to use any variations of that illustration. Anyhow please give me your thoughts and some good old advice. Thank you once again and have a most wonderful day.

Using the Library, web resources, and/or other materials, find a real-life application of a quadratic function. State the application, give the equation of the quadratic function, and state what the x and y in the application represent. Choose at least two values of x to input into your function and find the corresponding y for each. State, in words, what each x and y means in terms of your real-life application.

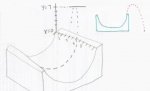
Lets just say that a special team of engineers have been deployed to develop the perfect half pipe doing aerial tricks when the roller – blader, biker, skate boarder etc… launch from either lip of a half pipe. They have decided to construct a 15 foot tall by 20 foot wide and 17 foot long half pipe for their test purposes after many hours of working this out on paper. The ramp has special tick marks in feet across the lip of both sides to determine the possible point of landing from the various aerial tricks performed. Therefore x is equivalent to tick marks across the lip of the ramp and y is equivalent to the amount of air or height reached. The y factor is important in order to develop an adequately sized back board for the ramp, so it isn’t to high or to low in case a grind along its edge was decided to be performed. Therefore using the following equation y=1x^2+2x-15 and our two x coordinates being x = 2 foot tick mark and x = the 10 foot tick mark we will determine how much air our test roller-blader achieved during his two runs.

y=1(2)^2+2(2)+0 y=1(5)^2+2(5)-15
y=4+4-15 y=25+10-15
y= 7ft y= 20ft

 

[mmm4444bot]

… x is equivalent to tick marks across the lip of the ramp and y is equivalent to the … height reached.

Whenever we impose an xy-coordinate system to describe a real-world situation, we always need to define the location of the origin.

I understand that the variable y represents some height, but it's not clear to me where y = 0 is located.

In other words, y represents the height in feet from where to where?

It seems to me that the variable x also represents some distance in feet, but, again, where is the origin?

In other words, when x = 10, then that represents 10 feet from where to where?

These two unresolved questions make it unclear to me whether or not the parabola represents the skater's trajectory OR the shape of the ramp.

If the "tick marks" run along the lip of the half pipe, then the x-axis is perpendicular to the direction of the skater. This does not make sense to me, so I must not understand what you're thinking.

Can you post an more detailed description of where you placed the axes when you imposed your coordinate system. Perhaps, an illustration would be even better.

-

The y factor is important … so [the backboard] isn’t [too] high or [too] low …

y = 1x^2 + 2x - 15

… x = 2 foot tick mark and x = the 10 foot tick mark …

Firstly, note that we do not write coefficients of 1 or -1.

If the coefficient on x^2 is 1, then we write x^2 instead of 1x^2.

If the coefficient on x^2 is -1, then we write -x^2 instead of -1x^2.

Secondly, your equation gives the following values.

When x = 2, y = -7.

When x = 10, y = 105.

What does negative seven feet mean in this model?

Can the backboard be 105 feet tall?

 

[adicus22]

I really appreciate the critique, you are very thorough in your explinations. However I suppose in this case that I am looking for yours or anyones opinion on whether this illustration seems to be feasible. Roller-blading is somethi g that I enjoy, especially the half pipe. It has been awhile but I know I still have it in me. Any how my focus is more so on the trajectory of the height of the skater from the point that they launch from the ramp to the point that they land. Would this not in fact be a representation of a quadratic function. Say skater X lauched from the 10ft ticek mark in the ramp, which would be the center and executed a 360 degree turn and landed, the y coordinate of intrest would be the maxium height that he reached before his descent. ldThe other point of interest would be for the application I'm using is, is there adequate room to conduct certain tricks without the skaters going over the edge or the back of the ramp.

My main problem with this is if I did use the equation for trajectory I don't understand how to determine my x and y coordinate. So instead of overcomplicating something that I don't grasp after exhausting several examples on the internet I decided to put things in terms I can relate to but I am still stumped to a degree.

I hope this sheds enough light for you to make enough sense of this to help me. Once a gain thank you.

 

[mmm4444bot]

… I am looking for [yours'] or [anyone's] opinion on whether this illustration seems to be feasible …

… my focus is more so on the trajectory of the height of the skater from the point that they launch from the ramp to the point that they land. Would this not in fact be a representation of a quadratic function. …

I'm still not sure that I have your picture accurately in my head.

Is the skater landing somewhere other than on the half pipe?

If the skater lands somewhere on the half pipe, then the path of flight is not a parabola; therefore, the height cannot be modeled with a quadratic polynomial.

You need a situation where the quantity y depends upon some other quantity x, such that y = ax^2 + bx + c.

I do not see how the height obtained depends upon the point of launch.

Once the skater leaves the half pipe, the height is a quadratic function of time. The parameters a, b and c are determined by various factors (eg: the force of gravity, the initial velocity, the initial height, the trajectory angle).

My gut feeling is that you've got something outside-the-box that cannot be modeled with a quadratic function.

I will upload a sloppy drawing of the picture in my head.

 

[Denis]

Re:

I will upload a sloppy drawing of the picture in my head.

S'that cause you too skateboarded at one time, and landed on your head too often ? :roll:

 

[mmm4444bot]

The red path is a parabola that shows the location of an object before it lands on the ground. (The height is a quadratic function of time.)

The pencil drawing is my guess at what you're talking about. Here, the x-values do not determine the y-values over the course of the trajectory (x and y both depend upon time, and the parameters come from other factors previously mentioned).

[attachment=0:17nm17hm]HalfPipe.JPG[/attachment:17nm17hm]

If I've grossly misunderstood your descriptions, then let me know.

If not, then continue thinking outside-the-box, as you please. But don't cross over the point of no return to the actual instructions that are given in this exercise, lest you find yourself at deadline with no quadratic relationship to show for your "research on the Internet".

 

[mmm4444bot]

… S'that cause you too skateboarded at one time, and landed on your head too often ?

Denis, I've told you multiple times that my head was born this way. The sign that you cannot remember this information presents as possible onset of organic deficit in your penthouse, as well.

I would suggest that you cancel your appointment with Dr. Cutie, post haste. Focus on the penthouse, before more bulbs burn out.

:twisted:

 

[mmm4444bot]

Click here to see an assignment that links skateboarding and parabolas.

Hints that come to my mind for other research are parabolic mirrors (a famous mathematician built one to burn enemy ships at sea), the outlet of nozzles used in firefighting (designed for things like customized water flow or spread); encryption and error-checking in computer science, intensity of light, intensity of signals, dynamically allocating and sharing bandwidth (Quality of Service [Qos]), vehicle suspension, the shape of a dam's spillway, the efficacy of AIDS drugs, earnings on Wall Street, statistics, and number theory.

If you consider optimization applications and quadratic regression, then models arise in practically every natural and social science.

There are literally tens of thousands of valid possibilities for this assignment.

 

[adicus22]

I give you a standing ovation. Your help has been most wonderful and very much appreciated. I am not one to just caressly toss my words around but am a firm believer in giving honor to whom it is due as the bible says. Funny thing is I haven't been online all this time I just got in from our evening church service and just forgot to log out. I reside down here in little old Graham WA, not too far from you. Anyhow you have a good evening and I hope you and Denis are able to work out your issues :lol: . Well gents enjoy and I'm sure you will be hearing from me again, I'll be sure to stock up on silver bullets.

 

[mmm4444bot]

You're welcome, but don't make me out to be too noble.

It's a coincidence that you live in Graham. I've been itching to drive to Long Beach for a few days' escape from my guards, and I've always wanted to check out the Harold E. LeMay Museum. I'm thinking of spending a night near there along the way because it must take several hours to view all those vehicles.

-

… I hope you and Denis are able to work out your issues …

I hope not. Life has so few pleasures.

 

[Denis]

Re:

Denis, I've told you multiple times that my head was born this way. The sign that you cannot remember this information presents as possible onset of organic deficit in your penthouse, as well.

I'll have you know that I have a very good memory, even if it's short :wink:

> Adicus: "I give you a standing ovation"
No chairs at your place?
Only "standing ovation" Mark ever gets is when he leaves town :twisted: