Agent Smith
Full Member
- Joined
- Oct 18, 2023
- Messages
- 462
So I was on a science forum and saw an interesting question. Take the equation y=−x.
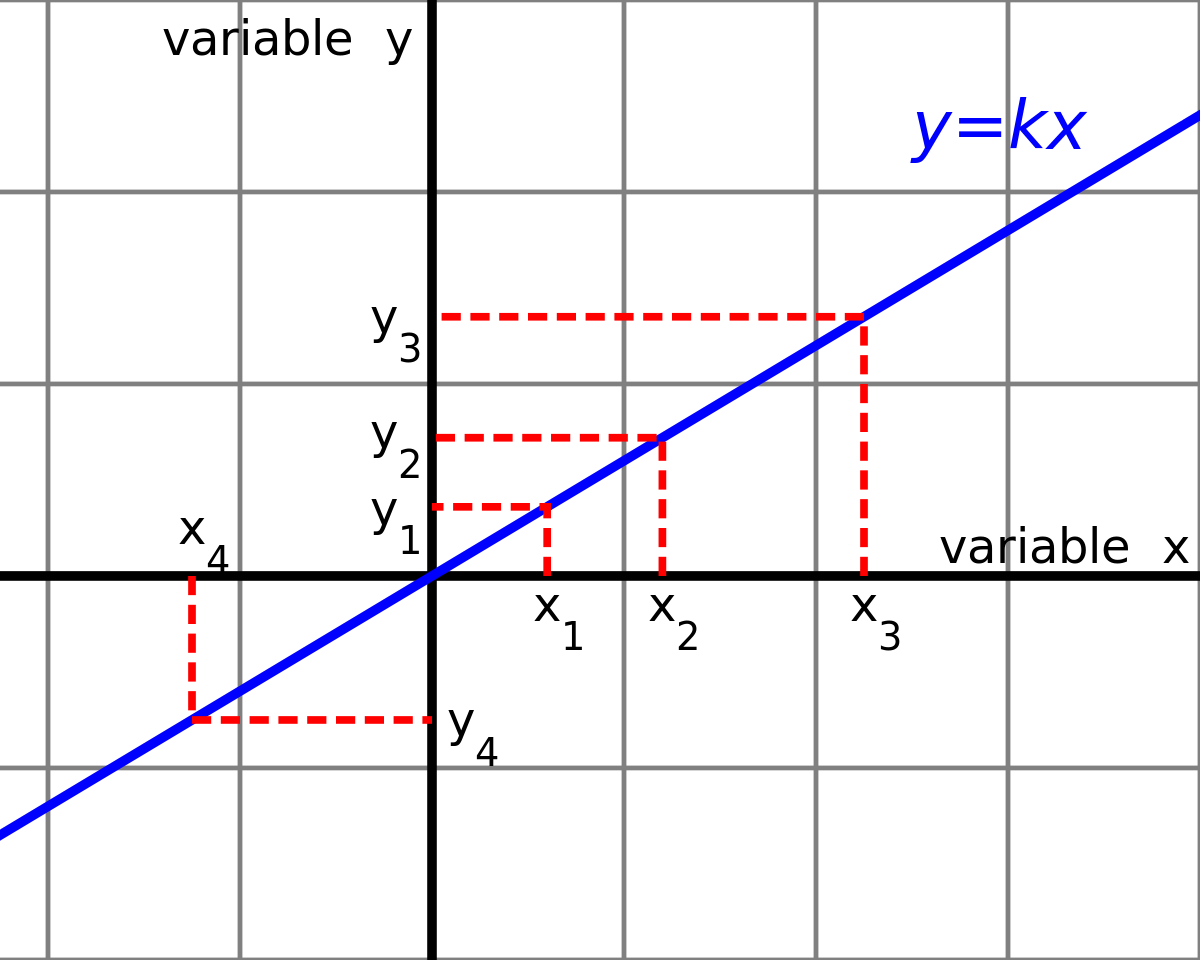
The slope is −1 and so as x increases y decreases and vice versa. This confused the poster, as does it me, because this is how inverse proportionality y∝x1 is also described.
How would you to clear this matter up?
The slope is −1 and so as x increases y decreases and vice versa. This confused the poster, as does it me, because this is how inverse proportionality y∝x1 is also described.
How would you to clear this matter up?