I was trying to solve the equation 0 = sqrt(x)/(15000000 ln(sqrt(x)/3))-1 for x, but i can't seem to do it. Wolfram says that i'm supposed to use something called the analytic continuation of the product log function or the Lambert W-Function. I'm in Calc 3 right now and i've never run into either of these ideas.
Product Log Function?
- Thread starter mathkid9
- Start date
Instead of trying this algebraically, just try a little trial and error.
\(\displaystyle \frac{\sqrt{x}}{15000000ln(\frac{\sqrt{x}}{3})}=1\)
\(\displaystyle \frac{\sqrt{x}}{ln(\frac{\sqrt{x}}{3})}=15000000\)
If we use x=3, we can see in the denominator we get ln(1)=0, which is undefined.
Therefore, for the number to be as large as 15,000,000 it has to be a wee bit more than 9.
This gives x=9.0000036000024
This is an approximation which gives a solution of .0000003
Close to 0.
\(\displaystyle \frac{\sqrt{x}}{15000000ln(\frac{\sqrt{x}}{3})}=1\)
\(\displaystyle \frac{\sqrt{x}}{ln(\frac{\sqrt{x}}{3})}=15000000\)
If we use x=3, we can see in the denominator we get ln(1)=0, which is undefined.
Therefore, for the number to be as large as 15,000,000 it has to be a wee bit more than 9.
This gives x=9.0000036000024
This is an approximation which gives a solution of .0000003
Close to 0.
unfortunately there are several different answers. 9 is pretty good, but unfortunately so is 31 trillion. i'm attempting to use this in a program i'm writing, but having run through it a few times with approximations, i'm instead getting an answer of about 80 quadrillion. sooooo.... yeah. not sure where to go from here. thanks for the help though.
Actually, no. x=31,000,000,000,000 gives 385,742.418773
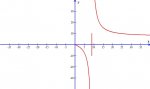
Graph \(\displaystyle \frac{\sqrt{x}}{ln(\frac{\sqrt{x}}{3})}\)
There is a vertical asymptote at x=9.
Your equation is \(\displaystyle \frac{\sqrt{x}}{ln(\frac{\sqrt{x}}{3})}=15,000,000\)
As \(\displaystyle x\to 9\), then the limit ----> infinity.
Since 15 million is quite large, this suggests the x value is close to 9 as we approach from the right.
Technically, there is no limit since when we approach from the left it goes off toward negative infinity.
Perhaps that is why your program will not work.
Graph \(\displaystyle \frac{\sqrt{x}}{ln(\frac{\sqrt{x}}{3})}\)
There is a vertical asymptote at x=9.
Your equation is \(\displaystyle \frac{\sqrt{x}}{ln(\frac{\sqrt{x}}{3})}=15,000,000\)
As \(\displaystyle x\to 9\), then the limit ----> infinity.
Since 15 million is quite large, this suggests the x value is close to 9 as we approach from the right.
Technically, there is no limit since when we approach from the left it goes off toward negative infinity.
Perhaps that is why your program will not work.
Attachments
actually, the program runs perfectly, its just for computing a maximum value before i get a memory error.
basically what the program does, is take a number, and compute all of the primes beneath that number, and write them to a file. dividing by every number, ie 1,2,3,4,5,etc, is redundant because dividing by 4 is alread covered by dividing by 2 etc., and in truth, you only need to divide by a list of already computed primes. so i have an array created in which to store the primes. the approximate distribution of primes at a given number is one prime ever ln(x) numbers. so to compute how many primes there should be in theory, i used ln(x/3), which gives about .1% error (for example between 1 and 50,000 there are 5133 primes, and 50,000/ln(50,000/3) gives 5143). so i created an array which has x/ln(x/3) values in it to maximize memory use. but to save even more memory, i realized that the only primes less than the sqrt of your highest number would have to be stored in the array. so for example if you were computing primes between 1 and 50,000, it doesnt matter that 49,999 is a prime for the array, because you would never have to divide 50,000 by 49,999 because it's parallel on the other side of the sqrt of 50,000 has already been tested. so instead, i creadted an array with sqrt(x)/ln(sqrt(x)/3) places in it, where x is the highest number, in this case 50,000. from my personal experience, i know that i can create an array of about 15,000,000 without having a memory error. so the maximum highest number i can compute to is defined by the equation 15,000,000=sqrt(x)/ln(sqrt(x)/3). from multiple runs of the program, i dont get a memory error until i input values in the range of 75-80 quadrillion, leading me to belive that the value of x that i'm looking for is in that range. unless that is, i had some huge lapse of logic which i'm missing.
basically what the program does, is take a number, and compute all of the primes beneath that number, and write them to a file. dividing by every number, ie 1,2,3,4,5,etc, is redundant because dividing by 4 is alread covered by dividing by 2 etc., and in truth, you only need to divide by a list of already computed primes. so i have an array created in which to store the primes. the approximate distribution of primes at a given number is one prime ever ln(x) numbers. so to compute how many primes there should be in theory, i used ln(x/3), which gives about .1% error (for example between 1 and 50,000 there are 5133 primes, and 50,000/ln(50,000/3) gives 5143). so i created an array which has x/ln(x/3) values in it to maximize memory use. but to save even more memory, i realized that the only primes less than the sqrt of your highest number would have to be stored in the array. so for example if you were computing primes between 1 and 50,000, it doesnt matter that 49,999 is a prime for the array, because you would never have to divide 50,000 by 49,999 because it's parallel on the other side of the sqrt of 50,000 has already been tested. so instead, i creadted an array with sqrt(x)/ln(sqrt(x)/3) places in it, where x is the highest number, in this case 50,000. from my personal experience, i know that i can create an array of about 15,000,000 without having a memory error. so the maximum highest number i can compute to is defined by the equation 15,000,000=sqrt(x)/ln(sqrt(x)/3). from multiple runs of the program, i dont get a memory error until i input values in the range of 75-80 quadrillion, leading me to belive that the value of x that i'm looking for is in that range. unless that is, i had some huge lapse of logic which i'm missing.
What if instead of sqrt of x, we denote x to be the sqrt of y. so we get:
x=sqrt(y)
then 15,000,000=x/ln(x/3), a much simpler equation
plugging this into wolfram gives two possible answers for x, specifically 3 and 275005000.
http://www.wolframalpha.com/input/?i=15 ... 28x%2F3%29
and if x^2=y, and x=3 and 275005000, then y=9 and 75.62775003 quadrillion. which is in the range i thought it would be.
x=sqrt(y)
then 15,000,000=x/ln(x/3), a much simpler equation
plugging this into wolfram gives two possible answers for x, specifically 3 and 275005000.
http://www.wolframalpha.com/input/?i=15 ... 28x%2F3%29
and if x^2=y, and x=3 and 275005000, then y=9 and 75.62775003 quadrillion. which is in the range i thought it would be.