problem with derivative

[red and white kop!]

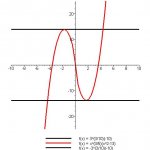
ok so for the function f(x) = (x^(3/5))(x^(2)-13) (the many parentheses are to make it clear) i am supposed to find f'(x) and the intervals in which f(x) is decreasing and increasing
i found f'(x) = (13/5)(x^(-2/5))(x^(2)-3)
i think this is correct
but then to find the increasing and decreasing intervals i got it wrong
i started with the increasing interval and got x>sqrt3 and -sqrt3>x>0, i keep doing it over and over but i dont understand where its wrong

 

[mmm4444bot]

Some of those parentheses are not needed.

f(x) = x^(3/5) * (x^2 - 13)

f`(x) = (13/5) * x^(-2/5) * (x^2 - 13)

±?3 define three intervals.

(-?, -?3) (-?3, ?3) (?3, ?)

You could pick a test value within each of these intervals, and then evaluate f`(x) at that point to see whether it's positive or negative.

 

[soroban]

Hello, red and white kop!

We are concerned with four intervals.

\(\displaystyle f(x) \:=\: x^{\frac{3}{5}} (x^2-13)\)

Find \(\displaystyle f'(x)\) and the intervals in which \(\displaystyle f(x)\) is decreasing and increasing

\(\displaystyle \text{We have: }\;f(x) \;=\;x^{\frac{13}{5}} - 13x^{\frac{3}{5}}\)

. . \(\displaystyle \text{Then: }\;f'(x) \;=\;\tfrac{13}{5}x^{\frac{8}{5}} - \tfrac{39}{5}x^{-\frac{2}{5}}\)

\(\displaystyle \text{There is a }vertical\text{ tangent when }\boxed{x = 0}\)



\(\displaystyle \text{There are }horizontal\text{ tangents when }f'(x) \,=\,0\!:\;\;\frac{13}{5}x^{\frac{8}{5}} - \frac{39}{5x^{\frac{2}{5}}} \;=\;0\)

\(\displaystyle \text{Multiply by }\frac{5x^{\frac{2}{5}}}{13}:\;\;x^2 - 3 \:=\:0 \quad\Rightarrow\quad \boxed{x \:=\:\pm\sqrt{3}}\)



\(\displaystyle \text{The "slope diagram" looks like this:}\)


. . \(\displaystyle \begin{array}{ccccccc} \nearrow && \searrow && \searrow && \nearrow \\ --- & \circ & --- & \circ & --- & \circ & --- \\ & \text{-}\sqrt{3} && 0 && +\sqrt{3} \end{array}\)


\(\displaystyle \text{Therefore }\;\boxed{\begin{array}{ccc}\text{Decreasing:} & (\text{-}\sqrt{3},0) \cup (0,\sqrt{3}) \\ \\[-3mm] \text{Increasing:} & (\text{-}\infty, \text{-}\sqrt{3}) \cup (\sqrt{3}, \infty) \end{array}}\)

 

[mmm4444bot]

Good call, Soroban. I should have done the exercise.

 

[BigGlenntheHeavy]

\(\displaystyle Here \ is \ the \ graph.\)

\(\displaystyle Note: \ f(x) \ = \ x^{3/5}(x^2-13), \ f(0) \ = \ 0, \ hence \ no \ vertical \ asymptote \ at \ x \ = \ 0,\)

\(\displaystyle ergo, \ decreasing \ for \ [-\sqrt3,\sqrt3]\)

[attachment=0d2dazxk]bbb.jpg[/attachmentd2dazxk]