PLEASE HELP

[Guest]

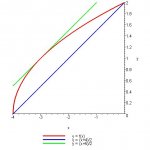
Consider the curve y^2 = 4 + x and chord AB joining the points A (-4,0) and B (0,2) on the curve.

a) Find the x and y coordinates on the point on the curve where the tangent line is parallel to chord AB

No idea what to do...

 

[mmm4444bot]

?

No idea what to do... Is this claim a gross exaggeration?

?
Perhaps, it's not yet clear that you need to determine the slope of chord AB, in this exercise.

This chord is a segment of the line passing through points A and B. (We call it a secant line.)

?
Do you know how to calculate a line's slope, given the coordinates of two points on that line ?

 

[Guest]

the slope is 1/2...then?
im still confused

 

[mmm4444bot]

?

Solve the given equation for y.

The given equation represents something called a conic section, in general. In particular, its graph is a parabola that opens to the right.

So, that graph is not the graph of a function, is it.

We need to solve for y, to get separate functions for the upper and lower halves of this "sideways" parabola.

Next up, determining which half contains points A and B, followed by calculating the first derivative of the function for that half.

 

[Guest]

oh wow! im slow xD

i uh...did this. so slope of AB is 1/2 correct?
and given y2 = 4 + x, its x = y2 - 4

x' = 2y => 2y = 1/2 => y = 1, x = -3

am i right? =D

 

[BigGlenntheHeavy]

\(\displaystyle y \ = \ \sqrt{4+x}, \ y' \ = \ \frac{1}{2\sqrt{4+x}} \ = \ m\)

\(\displaystyle A(-4,0) \ and \ B(0,2) \ \implies \ m \ = \ \frac{1}{2}, \ y \ = \ \frac{x+4}{2}\)

\(\displaystyle Hence, \ \frac{1}{2} \ = \ \frac{1}{2\sqrt{4+x}} \ \implies \ x \ = \ -3 \ \implies \ y \ = \ 1\)

\(\displaystyle m \ = \ \frac{1}{2}, \ point \ = \ (-3,1) \ \implies \ y \ = \ \frac{x+5}{2}\)

\(\displaystyle See \ graph\)

[attachment=0:1qd6ssct]123.jpg[/attachment:1qd6ssct]