Please Help with Calc!

[Guest]

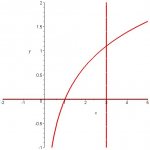
Let R be the region enclosed by the graph of y = lnx, the line x=3, and the x-axis. Use disks to find the volume of the sold generated by revolving region R about the x-axis.

So... would it be something like... the Integral(1->3)[lnx dx]?

if so...how would I calculate this? I'm very confused =[

 

[Guest]

ok...my last post for solving was retarded...so far i have this..

dv = pi*r2*dx

V = pi * integral(1->3)[(lnx)^2dx]

so... with integration of parts and stuff...i ended up with...

V = pi * [x[(lnx)^2 - 2xlnx + 2]](1->3)

am i on the right track?

 

[daon]

Your set up is correct.

 

[BigGlenntheHeavy]

\(\displaystyle \pi\int_{1}^{3}[ln|x|]^{2}dx \ \dot= \ 3.233, \ note; \ Use \ I \ by \ P \ twice \ after \ substituting \ k \ = \ ln|x|.\)

\(\displaystyle See \ graph.\)

[attachment=0:2a3xkgam]abc.jpg[/attachment:2a3xkgam]