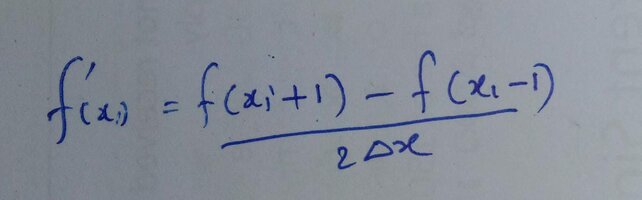Please help to solve this problem: u=f(x,y) satisfies d^2u/dx^2 + d^u/dy^2 = 0 in 0<x<1, 0<y<1, with...
- Thread starter PA3040D
- Start date
First, express δ2u/δx2 as an approximation using central-difference numerical approximation with a finite step length of 'h'
Next, express δ2u/δy2 as an approximation using central-difference numerical approximation with a finite step length of 'h'
Use the above into your original PDE. Continue.....
Then start with
express δu/δx as an approximation using central-difference numerical approximation with a finite step length of 'h'
Look up in your textbook/class-notes.
What is x1'? How/why is it different from x1?
Where is 'h' in the equation that you have presented?
As you know all x terms are same and h and delta x both are same
So apply this "knowledge" and rewrite the equation in response #6 - correctly.
Steven G
Elite Member
- Joined
- Dec 30, 2014
- Messages
- 14,561
That equality sign in the image is not valid!
Yes ... I got it thanks
I think now it is correct but my original problem is remaining same . Is there any one who have enough knowledge to answer my original question
Steven G
Elite Member
- Joined
- Dec 30, 2014
- Messages
- 14,561
You are correct about there being an approximate sign in the middle, but still you have a mistake. In fact, it is a very big mistake.
You are NOT paying attention to - what you are writing! Why would you want to approximate f(x) like that?
Ahhh yes f prime should come
This NOT an ONE step problem. You got:
f'(xi) = [f(xi+h) - f(xi-h)]/(2*h)
Now you have to write similar expression for f"(xi), which is of course [f'(xi)]'
Watch those ' and " - please consult your textbook/class-notes.
That really does not matter!
Are you enrolled in a class now where the topic of discussion is (or has been) - Numerical Analysis (numerical approximations of differential equations)?