PARAMETRIC EQUATIONS and TANGENT LINE
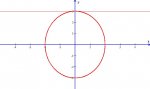
a) Sketch the curve with parametric equations: x=2cos2t, y=3sin2t, z=t. Indicate with an arrow the direction in which t increases. You do not have to sketch the curve but just state all information needed to sketch the curve nicely such as the traces, points of intersection, vertices axis etc. Please do not forget to indicate with the direction in which t increases (clockwise or counterclockwise direction).
b) Find parametric equations for the tangent line to the curve in question a) at the point (0,3, ?/4). Please note that if I say ?/4 I mean pi/4
I did try this problem but I was unable to complete it and please if you can solve it for me I will really appreciate it.
a) Sketch the curve with parametric equations: x=2cos2t, y=3sin2t, z=t. Indicate with an arrow the direction in which t increases. You do not have to sketch the curve but just state all information needed to sketch the curve nicely such as the traces, points of intersection, vertices axis etc. Please do not forget to indicate with the direction in which t increases (clockwise or counterclockwise direction).
b) Find parametric equations for the tangent line to the curve in question a) at the point (0,3, ?/4). Please note that if I say ?/4 I mean pi/4
I did try this problem but I was unable to complete it and please if you can solve it for me I will really appreciate it.