Ryan Rigdon
Junior Member
- Joined
- Jun 10, 2010
- Messages
- 246
The problem
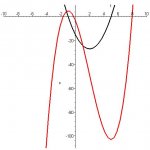
An object moves along a horizontal coordinate line in such a way that its position at time t is specified by
s = t^3 - 6t^2 - 15t - 3. Here s is measured in centimeters and t in seconds. When is the slowing down; that is,
when is its speed decreasing?
My work.
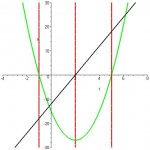
v = ds/dt = 3t^2 - 12t - 15 = 3(t^2 - 4t - 5) = 3(t-5)(t+1)
the velocity is t = 5, -1 when v=0
the velocity is positive v>0 when (t-5)(t+1)>0 , interval notation (-infinity , -1) U (5, infinity)
Our question was when is the speed decreasing?
v<0 (t-5)(t+1)<0
The objects speed is decreasing during the interval (5 , -1).
An object moves along a horizontal coordinate line in such a way that its position at time t is specified by
s = t^3 - 6t^2 - 15t - 3. Here s is measured in centimeters and t in seconds. When is the slowing down; that is,
when is its speed decreasing?
My work.
v = ds/dt = 3t^2 - 12t - 15 = 3(t^2 - 4t - 5) = 3(t-5)(t+1)
the velocity is t = 5, -1 when v=0
the velocity is positive v>0 when (t-5)(t+1)>0 , interval notation (-infinity , -1) U (5, infinity)
Our question was when is the speed decreasing?
v<0 (t-5)(t+1)<0
The objects speed is decreasing during the interval (5 , -1).