Hi
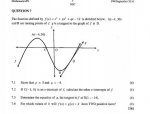
I am writing my final Mathematics exams for Grade 12 in South Africa in 5 days. I am well prepared with an aim of getting 100%, but one concept in functions might prevent that - the concept of how the nature of roots are affected by vertical/horizontal shifts in a function, and how to determine the values of the shift to obtain the required roots. I attach 3 example questions...
They are 8.1.4, 7.4 and 4.5
Please help me find Youtube Videos/Websites or any resource that might help me understand how to approach these questions.
Thanks

I am writing my final Mathematics exams for Grade 12 in South Africa in 5 days. I am well prepared with an aim of getting 100%, but one concept in functions might prevent that - the concept of how the nature of roots are affected by vertical/horizontal shifts in a function, and how to determine the values of the shift to obtain the required roots. I attach 3 example questions...
They are 8.1.4, 7.4 and 4.5
Please help me find Youtube Videos/Websites or any resource that might help me understand how to approach these questions.
Thanks