Maximization of Area

[mjfuentes85]

So I have this word problem on trying to maximize area of a box, so i know i am going to have to find an equation where I set the derivative of that equation to zero and solve for my x values and y values to come up w an equation that specifics the max. But i am kind of stuck on this problem any help is appreciated.
Heres the problem :

A metal box with a square base and no top holds 1000 cubic centimeters. It is formed by folding up the sides of the flattened pattern pictured here and seaming up four sides. ( The picture shows a rectangle with an a joining rectangle at each side). The material of the box costs $1.00 per square meter and the cost to seam the sides is 5 cents per meter. Find the dimensions of the box that costs the least amount to produce.

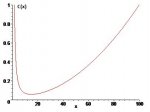
I need to include the function i am trying to minimize, the domain, graph of the function, derivative and critical points, and proof that i have found a minimum. Again any help is appreciated. thnx in advance.
-Marcus

 

[tkhunny]

Well, do it. Start by introducing suitable notation.

x = side of the square base of the box.
h = height of the box.

Now we can talk about stuff.

Base Area of Box = x^2
Lateral area of box = 4 * x * h
Volume of Box = h * x^2

How much must be stiched? Can you state it in terms of h and x?

There is no reason why you cannot ALWAYS do what just happened. You NEVER need to have no idea where to start.

 

[mjfuentes85]

The information i have provided is the extent of all the information i have. I failed to mention that this is a computer lab so i am going to say that the amount stitched is going to be in terms of x and y. what can i do with the values of 1.00 per square meter and 5 cents per meter ??

 

[mmm4444bot]

i am going to say that the amount stitched is going to be in terms of x and y.

No, it's in terms of y only.

We understand that you're changing the name h to y. That's okay.

x = base side length

y = box height



what can i do with the values of 1.00 per square meter and 5 cents per meter ??

First, you need to use a relation between x and y to express y in terms of x.

Then use the given dollar amounts with the variable x to write mathematical expressions representing "Total Material Cost" and "Total Stitching Cost" because:

Total Manufacturing Cost = Total Material Cost + Total Stitching Cost

and that's what you're trying to minimize, yes?

In other words, you eventually have to define f(x) = Total Material Cost + Total Stitching Cost

BTW, the posted exercise does not involve maximizing the volume of the box (or area or anything else); you're asked to minimize the cost to produce the box. The volume of the box is fixed at 1000 cm^3.

You seem somewhat turned around.

Let's deal with the first part of this exercise. In order to write a function definition for f(x) that outputs the total manufacturing cost in terms of x, we need to know y in terms of x. We rewrite y in terms of x using the volume equation.

Can you write an equation in x and y that shows the volume to be 1000 cm^3 ? If so, then solve it for y.

If you don't know the formula for calculating the volume of a rectangular box, let us know.



My edits: Fixed typographical errors with units. Later, used red crayon.

 

[mmm4444bot]

I returned because I realized that the given units for the volume are cm^3 and the units for the costs are per m and m^2.

You need to decide whether you want to report the dimensions of the box in terms of centimeters or meters. What's your choice?

(My gut feeling is to report the box dimensions in centimeters, leaving the volume as 1000 cm^3, but converting $1/m^2 to the equivalent dollars per cm^2 and converting 5 cents per m to the equivalent dollars per cm.)



My edit: changed cents/cm to dollars/cm

 

[tkhunny]

The information i have provided is the extent of all the information i have. I failed to mention that this is a computer lab so i am going to say that the amount stitched is going to be in terms of x and y. what can i do with the values of 1.00 per square meter and 5 cents per meter ??

This makes no sense. What does a computer lab have to do with suitable notation? You understand it or you don't. Call them Steve and Bob, if you like. It is the understanding that matters, not the nomenclature.

If you insist on using x and y, which would have NOTHING to do with being a computer lab, then please WRITE DOWN those definitions.

x = what?
y = what?

 

[mmm4444bot]

It's been awhile, since we gave you some guidance. Perhaps you found help elsewhere and finished this exercise. For the benefit of future readers of this thread, I'll complete the exercise as an example for this type of problem.

I choose to report the box dimensions in centimeters, so the given cost information needs unit conversions.

1 square meter (m^2) = 10000 square centimeters (cm^2)

1 meter (m) = 100 centimeters (cm)

$1.00/m^2 is equivalent to $1.00/10000 cm^2

$0.05/m is equivalent to $0.05/100 cm

Hence, we have the following cost information: materials cost 0.0001 dollars per cm^2 and seaming costs 0.0005 dollars per cm.

x = side length of the base (in cm)

y = height of the finished box (in cm)

Volume (in cm^3) = (width)(length)(height)

1000 = x^2 y

Solving this equation for y in terms of x yields y = 1000/x^2

To create a cost function C(x), we need to determine expressions in x that represent both the total area and the total seaming length.

The area consists of a square (x^2) and four rectangles (each xy).

Total Area = x^2 + 4x(1000/x^2) = x^2 + 4000/x

The seams consist of the height at all four edges of the finished box (4y).

Total Seams = 4(1000/x^2) = 4000/x^2

The total cost function is the sum of (material area)(cost per cm^2) + (seams)(cost per cm)

Using our expressions, we have (x^2 + 4000/x)(0.0001) + (4000/x^2)(0.0005)

C(x) = 0.0001x^2 + 0.4/x + 2/x^2

The restricted domain of this function is x > 0.

[attachment=0:39y9af0w]boxcost.JPG[/attachment:39y9af0w]

Use the Power Rule, to differentiate term-by-term.

C`(x) = 0.0002x - 0.4/x^2 - 4/x^3

Solving the equation C`(x) = 0 yields one critical point within the restricted domain of C: x = 14.9453 (rounded).

This is the value of x where C(x) is smallest (i.e., the minimum cost to manufacture the box).

y = 1000/14.9453^2 = 4.4770

The finished box which costs the least to manufacture measures roughly 14.9 cm by 14.9 cm by 4.5 cm

Do your answers match mine?

Cheers