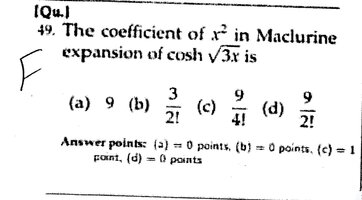YehiaMedhat
Junior Member
- Joined
- Oct 9, 2022
- Messages
- 74
The question is: The coeffiecient of x2 in Maclurine expansion of cosh(3x) is ...
So, trying to solve the question I have to diffrentiate, don't I? so, the first deriviative is 23x3sinh(3x), and as it's Maclurine expansion how would I insert the value of x=0 to the deriviative? Is there's a mistake I have done?
So, trying to solve the question I have to diffrentiate, don't I? so, the first deriviative is 23x3sinh(3x), and as it's Maclurine expansion how would I insert the value of x=0 to the deriviative? Is there's a mistake I have done?