Length of the cardioid r = 5(1 - cos?) between ?=0, ?=?
- Thread starter MAC-A-TAC
- Start date
arc length in polar is \(\displaystyle L=\int_{\alpha}^{\beta}\sqrt{r^{2}+\left(\frac{dr}{d\theta}\right)^{2}}d{\theta}\)
Let's do the general case: \(\displaystyle a(1-cos{\theta})\)
\(\displaystyle r^{2}+\left(\frac{dr}{d\theta}\right)^{2}=[a(1-cos{\theta})]^{2}+[asin{\theta}]^{2}=4a^{2}sin^{2}(\frac{\theta}{2})\)
\(\displaystyle \int_{0}^{\pi}[2asin(\frac{\theta}{2})]d{\theta}\)
After you integrate and have the solution(it is very basic), note that you have a=5
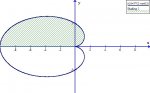
Here is the region you want the arc length of:
Let's do the general case: \(\displaystyle a(1-cos{\theta})\)
\(\displaystyle r^{2}+\left(\frac{dr}{d\theta}\right)^{2}=[a(1-cos{\theta})]^{2}+[asin{\theta}]^{2}=4a^{2}sin^{2}(\frac{\theta}{2})\)
\(\displaystyle \int_{0}^{\pi}[2asin(\frac{\theta}{2})]d{\theta}\)
After you integrate and have the solution(it is very basic), note that you have a=5
Here is the region you want the arc length of:
Attachments
There is no identities to use. Just factor out a 2.
Just do as the formula says.
I am going to use t instead of theta. No biggie, just easier.
\(\displaystyle r^{2}=25(1-cos(t))^{2}\)
\(\displaystyle \left(\frac{dr}{dt}\right)^{2}=25sin^{2}(t)\)
\(\displaystyle \sqrt{r^{2}+\left(\frac{dr}{dt}\right)^{2}}=5\sqrt{2(1-cos(t))}\)
There it is. But what you have does not correspond to what you have in your heading, \(\displaystyle 5\sqrt{(1-cos(t))}\)
The first one is correct. You forgot the 2 in the second one. See?.
But, \(\displaystyle \sqrt{1-cos(t)}\) is difficult to integrate. That is why we manipulate it so we can integrate as I showed in my first post.
BTW, it would help to use proper grouping symbols. What you have is \(\displaystyle 10\sqrt{1}-\frac{cos{\theta}}{2}\)
Just do as the formula says.
I am going to use t instead of theta. No biggie, just easier.
\(\displaystyle r^{2}=25(1-cos(t))^{2}\)
\(\displaystyle \left(\frac{dr}{dt}\right)^{2}=25sin^{2}(t)\)
\(\displaystyle \sqrt{r^{2}+\left(\frac{dr}{dt}\right)^{2}}=5\sqrt{2(1-cos(t))}\)
There it is. But what you have does not correspond to what you have in your heading, \(\displaystyle 5\sqrt{(1-cos(t))}\)
The first one is correct. You forgot the 2 in the second one. See?.
But, \(\displaystyle \sqrt{1-cos(t)}\) is difficult to integrate. That is why we manipulate it so we can integrate as I showed in my first post.
BTW, it would help to use proper grouping symbols. What you have is \(\displaystyle 10\sqrt{1}-\frac{cos{\theta}}{2}\)
