btw, I just edit my post above two times, but there is no "edited time stamp" (I mean, I don't see one)
In my experience, you can edit within 30 minutes, and if you do so soon enough (I don't know the definition), it doesn't mark that it was edited, but if you wait more than a few minutes it typically does.
(I edited it after 2 minutes to see what happens.)
(There was no edited date. I'm adding this after 5 minutes.)
but their examples include this
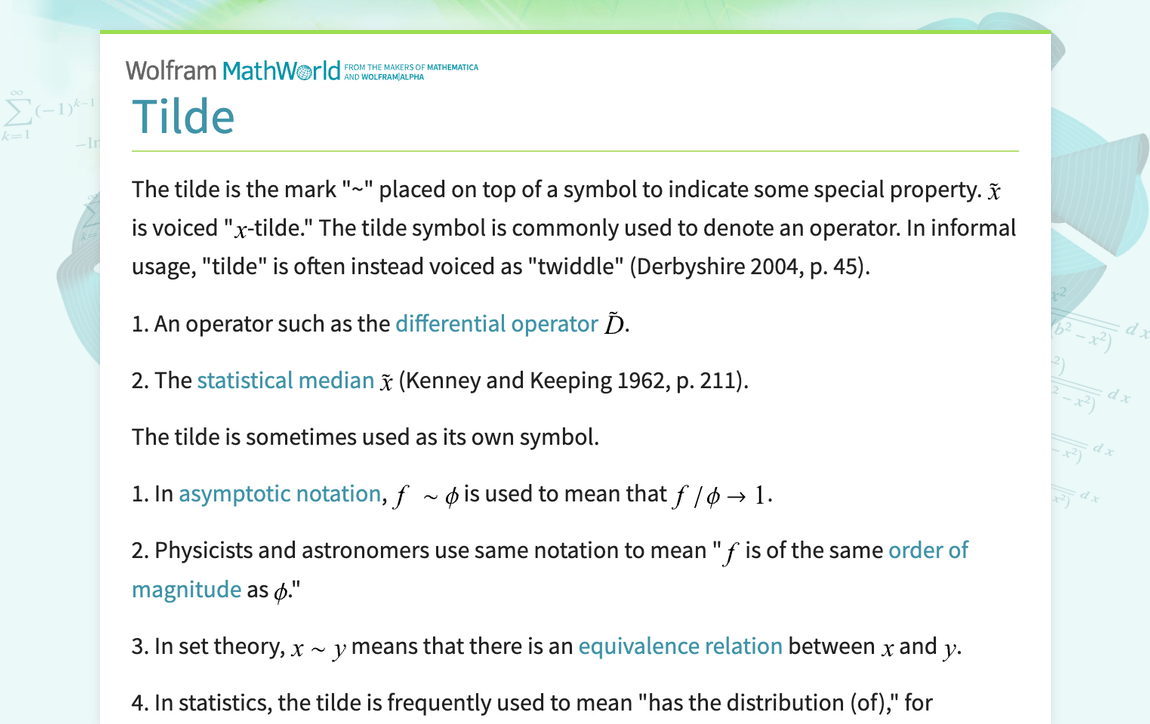
\(\displaystyle \bigg(2-\frac{\text{d}}{\text{dx}}\bigg)^n\)
and that looks strange. If d/dx is numerical amount, then why describe d/dx as "operator".
You're referring to an example at
https://mathworld.wolfram.com/DifferentialOperator.html, right?
The differential operator satisfies the identity
[imath]\left(2x-\dfrac{d}{dx}\right)^n 1=H_n(x)[/imath]
where [imath]H_n(x)[/imath] is a Hermite polynomial.
So you copied it wrong, though that doesn't make a big difference.
They demonstrate after this how the operator is applied; did you try following that?
Here's the idea, in more detail: [math]\left(2x-\dfrac{d}{dx}\right)^2 1=\\\left(2x-\dfrac{d}{dx}\right)\left(2x-\dfrac{d}{dx}\right) 1=\\\left(2x-\dfrac{d}{dx}\right)\left(2x\cdot1-\dfrac{d}{dx} 1\right)=\\\left(2x-\dfrac{d}{dx}\right)\left(2x\right)=\\2x\cdot2x-\dfrac{d}{dx}2x=\\4x^2-2[/math]
In effect, you pretend that [imath]\frac{d}{dx}[/imath] is just a "number" you are "multiplying" by, until you actually carry it out as a differentiation.
It's an operator because it does something to what follows, in this case by differentiating a function.
When used as a differential, symbol dx is infinitesimally small number. Then, it's not an operator. I'm not sure what their example means by subtracting d/dx instead of dx.
No, you seem to be confusing [imath]dx[/imath], which is a differential (for some purposes thought of as a small number) and [imath]\frac{d}{dx}[/imath], which operates on a function. The latter is an operator; the former is not.