Largest Value of m?

[the red baron]

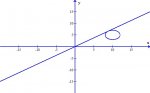
What is the largest value of m such that the graph of the equation y=mx meets the graph of the equation (x-10)^2 + (y-5)^2 = 4

I've been having a lot of trouble with this problem for the past 2 hours. Any help would be greatly appreciated.

 

[galactus]

Notice what you have here?. A circle with center at (10,5) with radius 2. The line y=mx passes through the origin.
Here's a start, see what happens if x=44/5.

 

[soroban]

Hello, the red baron!

Find the largest value of $\displaystyle m$ such that the graph of the equation: $\displaystyle y=mx$ .[1]
meets the graph of the equation: $\displaystyle (x-10)^2 + (y-5)^2 \:=\: 4$ .[2]

We have a line through the Origin and a circle: center (10,5), radius 2.

The line will be tangent to the circle.
. . Hence, their graphs will intersect at one point.

Substitute [1] into [2]: .$\displaystyle (x-10)^2 + (mx-5)^2 \:=\:4$

. . which simplifies to the quadratic: .$\displaystyle (m^2+1)x^2 - 10(m+2)x + 121 \:=\:0$

$\displaystyle \text{Quadratic Formula: }\;x \;=\;\frac{10(m+2) \pm\sqrt{100(m+2)^2 - 484(m^2+1)}}{2(m^2+1)}$


If there is one point of intersection, the discriminant is zero.

. . $\displaystyle 100(m+2)^2 - 484(m^2+1) \:=\:0$

. . $\displaystyle \text{which simplifies to: }\;96m^2 - 100m + 21 \:=\:0$

. . $\displaystyle \text{which factors: }\;(24m-7)(4m-3) \:=\:0$

. . $\displaystyle \text{and has roots: }\;m \;=\;\frac{7}{24},\;\frac{3}{4}$ . There are two tangents to the circle.


$\displaystyle \text{Therefore, the largest value of }m\text{ is: }\:m \:=\:\frac{3}{4}$

 

[the red baron]

Wow, thanks for all the great help. I actually get this now thanks to you guys.