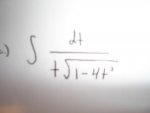inverse trig integration

[NRS]

Okay, I'm having a tough time with this problem, I really don't know where to start.

My main trouble is in getting the variable out of the denominator, I know it wil probobly be very obvious once I get help .
[attachment=0:1fpp5f1q]math.jpg[/attachment:1fpp5f1q]

 

[Aladdin]

---------------------------

When you take the radical symbol it's actually to the power 1/2 . . .

$\displaystyle \sqrt{x} = x^{1/2}$

 

[NRS]

Well, I'm still experiencing mental block, sorry. Why does it matter that the radical can be rewritten?

 

[Aladdin]

---------------------------

When you take the radical symbol it's actually to the power 1/2 . . .

$\displaystyle \sqrt{x} = x^{1/2}$

$\displaystyle \frac{1}{\sqrt{x}} = \ x^{-1/2}$

 

[BigGlenntheHeavy]

$\displaystyle \int\frac{dt}{\sqrt{1-4t^{2}}}. \ Let \ u \ = \ 2t, \ \implies \ du \ = \ 2dt$

$\displaystyle Hence, \ we \ have: \ \frac{1}{2}\int\frac{du}{\sqrt{1-u^{2}}} \ = \ \frac{arcsin|u|}{2}+C \ = \ \frac{arcsin|2t|}{2}+C$

 

[NRS]

$\displaystyle \int\frac{dt}{\sqrt{1-4t^{2}}}. \ Let \ u \ = \ 2t, \ \implies \ du \ = \ 2dt$

$\displaystyle Hence, \ we \ have: \ \frac{1}{2}\int\frac{du}{\sqrt{1-u^{2}}} \ = \ \frac{arcsin|u|}{2}+C \ = \ \frac{arcsin|2t|}{2}+C$

Did you leave out the t in the denominator?

 

[NRS]

Thankyou, I'll have to follow that later. Right now my sister's in line for the computer... so I'm going to have to wait to try my understanding on you're explanation, But I'm sure it will help

 

[BigGlenntheHeavy]

$\displaystyle OK, \ my \ mistake, \ thought \ t \ was \ a \ + \ sign, \ ergo \ one \ way:$

$\displaystyle \int\frac{dt}{t(1-4t^{2})^{1/2}}, \ Let \ u \ = \ (1-4t^{2})^{1/2} \ \implies \ t^{2} \ = \ \frac{1-u^{2}}{4}$

$\displaystyle and \ du \ = \ \frac{1}{2}(1-4t^{2})^{-1/2}(-8t)dt \ = \ \frac{-4t}{u}dt \ \implies \ dt \ = \ \frac{u}{-4t}du$

$\displaystyle Hence, \ \int\frac{dt}{t(1-4t^{2})^{1/2}} \ = \ \frac{-1}{4}\int\frac{u}{t^{2}u}du \ = \ \frac{-1}{4}\int\frac{du}{t^{2}} \ = \ -\int\frac{du}{1-u^{2}}.$

$\displaystyle = \ -\int\bigg[\frac{1}{2(u+1)}-\frac{1}{2(u-1)}\bigg]du \ = \ \frac{1}{2}\int\frac{du}{u-1}-\frac{1}{2}\int\frac{du}{u+1},$

$\displaystyle = \ (after \ resub, \ etc.) \ \frac{1}{2}ln\bigg|\frac{(1-4t^{2})^{1/2}-1}{(1-4t^{2})^{1/2}+1}\bigg|, \ Note: \ Absolute \ value \ sign \ is \ necessary.$

$\displaystyle Check: \ D_t\bigg[\frac{1}{2}ln\bigg|\frac{(1-4t^{2})^{1/2}-1}{(1-4t^{2})^{1/2}+1}\bigg|\bigg] \ = \ \frac{1}{t(1-4t^{2})^{1/2}}$

 

[BigGlenntheHeavy]

$\displaystyle Another \ way \ (probably \ the \ easiest):$

$\displaystyle \int\frac{dt}{t(1-4t^{2})^{1/2}}, \ Let \ 2t \ = \ sin(\theta) \ \implies \ t \ = \ \frac{sin(\theta)}{2} \ and \ dt \ = \ \frac{cos(\theta)}{2}d\theta.$

$\displaystyle Hence, \ we \ have \ \int\frac{cos(\theta)}{sin(\theta)cos(\theta)}d\theta \ = \ \int csc(\theta)d\theta \ = \ -ln|csc(\theta)+cot(\theta)|+C$

$\displaystyle Hence, \ we \ have \ \int\frac{dt}{t(1-4t^{2})^{1/2}} \ = \ -ln\bigg|\frac{1+(1-4t^{2})^{1/2}}{2t}\bigg|+C, \ -1/2 \ \le \ \ t \ \le \ 1/2, \ t \ \ne \ 0.$

$\displaystyle Check: \ D_t\bigg[-ln\bigg|\frac{1+(1-4t^{2})^{1/2}}{2t}\bigg|+C\bigg] \ = \ \frac{1}{t(1-4t^{2})^{1/2}}.$

 

[Aladdin]

Glenn I've tested my professor with this He ran away from the answer and said that it doesn't have an answer unless a boundry is exist . . .

 

[BigGlenntheHeavy]

$\displaystyle Right \ Aladdin, \ look \ at \ the \ integral. \ If \ we \ let f(x) \ = \ \frac{1}{t(1-4t^{2})^{1/2}},$

$\displaystyle then \ (1-4t^{2}) \ >0, \ Why? \ This \ implies \ 4t^{2} \ < \ 1, \ implies \ t^{2} \ < \ \frac{1}{4},$

$\displaystyle implies \ |t| \ < \ \frac{1}{2}, \ hence \ -\frac{1}{2} \ < \ t \ < \ \frac{1}{2}, \ t \ \ne \ 0$

$\displaystyle So, \ the \ solution \ is \ correct \ providing \ it \ is \ within \ this \ domain.$

$\displaystyle Just \ remember \ Aladdin, \ division \ by \ zero \ is \ a \ no-no.$

 

[Aladdin]

Ahaaa . . .

Thanks Glenn that was a nice prove . . .

Back to you question : You can't have a negative number under a radical in R .

 

[BigGlenntheHeavy]

$\displaystyle Look, \ \sqrt{1-4t^2}, \ then \ 1-4t^2 \ \ge \ 0 \ usually.$

$\displaystyle However \ if \ \sqrt{1-4t^{2}} \ is \ the \ denominator \ of \ a \ fraction, \ then \ {1-4t^{2}} \ > \ 0$

$\displaystyle as \ if \ it \ equal \ zero, \ then \ we \ would \ have \ division \ by \ zero, \ a \ no-no.$

 

[BigGlenntheHeavy]

$\displaystyle Another \ thing \ Aladdin, \ we \ were \ given \ to \ find \ the \ integral \ of \ a \ function, \ which \ we \ did.$

$\displaystyle The \ problem \ said \ nothing \ about \ the \ domain \ in \ which \ this \ integral \ will \ work.$

$\displaystyle You \ professor \ is \ wrong: \ as \ we \ were \ ask \ to \ find \ the \ antiderivative \ of \ f(x), \ not \ its \ domain$

$\displaystyle \ or \ range.$

 

[Aladdin]

$\displaystyle You \ professor \ is \ wrong: \ as \ we \ were \ ask \ to \ find \ the \ antiderivative \ of \ f(x), \ not \ its \ domain$

$\displaystyle \ or \ range.$

So the antideriative exist . . .

Yes , we're not asked to find the domain or range : :

So I'm going to give him your answer and see what he'll say !

 

[BigGlenntheHeavy]

$\displaystyle Here \ is \ the \ proof; \ send \ it \ to \ your \ professor.$

$\displaystyle \int\frac{dt}{t(1-4t^{2})^{1/2}} \ = \ -ln\bigg|\frac{1+(1-4t^{2})^{1/2}}{2t}\bigg|+C$
$\displaystyle Check: \ D_t\bigg[-ln\bigg|\frac{1+(1-4t^{2})^{1/2}}{2t}\bigg|+C\bigg] \ = \ \frac{1}{t(1-4t^{2})^{1/2}}.$

 

[daon]

As Glenn pointed out, this is a function with domain (-1/2,0)U(0,1/2). That is as general as it gets for this f in the Reals. Glenn's solution is the anti-derivative for f on any subset of this domain. The Definite Integral will converge for any bounds, say a to b, in the domain of the function as long as a and b have the same sign. The non-removable discontinuity at 0 is what might be causing the fuss with your professor.