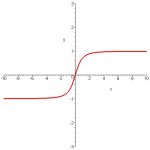
Given the function f defined by f(x)=1+x2x
1) Show that f admits an inverse over R .
2) Determine the Domain of the inverse .
3) Express the inverse function .
Work :
A function admits an inverse in it's domain if it is continuous , defined and strictly monotonic .
f(x) is always cont. def. in R
f(x) can be written (x)(1+x^2)^-1/2
f'(x) = (x)(-1/2)(1+x^2)(2x) + (1)(1+x^2)^-1/2
f'(x) = (x^2)(1+x^2) + 1/sqrt{1+x^2)
f'(x) is always positive on R . So it's stricly increasing .
So f(x) admits an invers .
2) Domain of invers = range f(x)
R= ]-inf , +inf[
f^-1 = f(R) = ]f(-inf) , f(+inf)[ = ] ? , ? [
I think there's a trick to be done here . I've took x^2 as a common factor but still ....
3)f^-1 = ?
y=x/sqr{1+x^2}
y^2=x^2/1+x^2
y^2*(1+x^2) = x^2
y^2 + y^2*x^2 = x^2
y^2 = x^2(-y^2+1)
x^2= y^2/(-y^2+1)
x = +- y(/sqr{1}{-y^2+1) ....
Thanks in advance .
1) Show that f admits an inverse over R .
2) Determine the Domain of the inverse .
3) Express the inverse function .
Work :
A function admits an inverse in it's domain if it is continuous , defined and strictly monotonic .
f(x) is always cont. def. in R
f(x) can be written (x)(1+x^2)^-1/2
f'(x) = (x)(-1/2)(1+x^2)(2x) + (1)(1+x^2)^-1/2
f'(x) = (x^2)(1+x^2) + 1/sqrt{1+x^2)
f'(x) is always positive on R . So it's stricly increasing .
So f(x) admits an invers .
2) Domain of invers = range f(x)
R= ]-inf , +inf[
f^-1 = f(R) = ]f(-inf) , f(+inf)[ = ] ? , ? [
I think there's a trick to be done here . I've took x^2 as a common factor but still ....
3)f^-1 = ?
y=x/sqr{1+x^2}
y^2=x^2/1+x^2
y^2*(1+x^2) = x^2
y^2 + y^2*x^2 = x^2
y^2 = x^2(-y^2+1)
x^2= y^2/(-y^2+1)
x = +- y(/sqr{1}{-y^2+1) ....
Thanks in advance .