inflection points

[ryan1015]

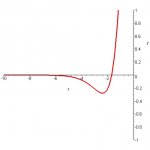
find the inflection points of the curve y=(4x^3 - x^2 -7x+10) e^2x correct to 6 decimal places


Natural logs really throw me off. my first derivative was (12X^2 - 2x -7)(e^2x) + (4x^3 - X^2 -7x +10)(2e^x)

my 2nd derivative was (24x -2)(e^2x) + (12x^2 - 2x -7)(2e^x) + (12x^2 -2x -7)(2e^x) + (4x^3 - X^2 -7x +10) (e^x) from there i am confused

 

[tkhunny]

Sorry, you were confused earlier than that.

\(\displaystyle \frac{d}{dx}e^{2x} = 2e^{2x}\) It's not a polynomial. Don't try to use the polynomila / power rule

Try that first derivative again and then do some algebra.

 

[BigGlenntheHeavy]

\(\displaystyle Here \ is \ the \ graph \ of \ f(x), \ does \ it \ tell \ you \ anything \ around \ x \ = \ -3?\)



[attachment=0:3kb0qwyj]ghi.jpg[/attachment:3kb0qwyj]