inflection point

[Mickey]

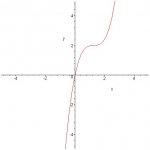
How do you find the inflection point of a graph of an expression that is a third degree polynomial that is constantly increasing. The graph of this polynomial goes through (0,0) and levels off as it goes upward to the right at about 2, then goes up more steeply again.

 

[galactus]

Can you post the graph?. That would help. Are you just given a graph and no expression?.

The second derivative of a cubic polynomial is linear.

To find the inflection points, try setting the second derivative equal to 0 and solving for x.

That is just some advice without knowing much else.

 

[Mickey]

Only a graph is given and therefore it's not possible to set the expression to 0. I don't know how to create a graph on this forum.

 

[galactus]

If you have the graph saved on your computer, try posting it via the 'upload attachments' link directly under the posting screen.

 

[BigGlenntheHeavy]

\(\displaystyle Let \ f(x) \ = \ (x-2^{1/3})^{3}+2\)

\(\displaystyle Then f '(x ) \ = \ 3(x-2^{1/3})^{2}\)

\(\displaystyle f "(x) \ = \ 6(x-2^{1/3})\)

\(\displaystyle Hence \ the \ inflection \ point \ is \ (2^{1/3},2)\)

[attachment=0:2ow1apa8]jak.jpg[/attachment:2ow1apa8]

 

[Mickey]

i
i
i
i i i
i
+ i
i
i
i
i
i
i

I had trouble uploading the pic I created of the graph so I'm showing it like this with the '+' represesenting the crossing of the x and y axes and the 'i's standing for the shape of the graph. Sorry, but I'm stuck uploading my image.

 

[Mickey]

BigGlenntheHeavy,

Where'd you get that expression from? The graph is virtually perfect by the way/

 

[BigGlenntheHeavy]

Just followed your instructions, is cubic, goes through (0,0), and relaxes at y = 2.

 

[Mickey]

What if it relaxed at 2.7? Does 2.7 go exactly where both 2^1/3 and 2 went in the expression?

 

[BigGlenntheHeavy]

\(\displaystyle Then \ f(x) \ = \ (x-2.7^{1/3})^{3}+2.7.\)

 

[Mickey]

Thanks ....great.