Implicit differentation

[crouchjr]

Anyone know how to do this problem?
Use implicit differentiation to find the slope of the tangent line to the curve

-2x^2-1xy-4y^3=-110

at the point (-2,3)

What does m=?

 

[tkhunny]

Step #1 Make sure the point is on the curve. Please demonstrate this.

Step #2 Use your Implicit Differentiation skills. This requires a nice understanding fo the Chain Rule

For the first term, I get -4x <== Just the good old polynomial rule there.

For the second term, I get -x*(dy/dx) - y <== A thing of beauty. Product rule and chain rule.

You do the third term and the other side of the equal sign.

Step #3 Find dy/dx

Step #4 Evaluate dy/dx at the given point (assuming it is on the curve).

 

[crouchjr]

12y^3 (dy/dx)=dy/dx(-110)=0 ?

 

[tkhunny]

Close. How about \(\displaystyle -12y^{2} \cdot \frac{dy}{dx}\). You missed th esign and the exponent.

Great. Now put it all together and find an expression for dy/dx.

Wait! Did you check to see that the point IS on the curve?

 

[BigGlenntheHeavy]

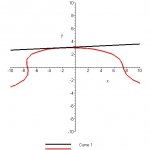
\(\displaystyle Given: \ -2x^{2}-xy-4y^{3}+110 \ = \ 0, \ and \ point(-2,3)\)

\(\displaystyle Find \ line \ tangent \ to \ f(x,y) \ at \ the \ point.\)

\(\displaystyle Implicit \ differentiation \ yields: \ -4x-[y+xy']-12y^{2}y'+0 \ = \ 0\)

\(\displaystyle -4x-y-xy'-12y^{2}y' \ = \ 0 \ \implies \ y' \ = \ -\frac{4x+y}{x+12y^{2}}, \ at \ (-2,3), \ m \ = \ \frac{5}{106}.\)

\(\displaystyle Hence, \ y-3 \ = \ \frac{5}{106}(x+2), \ y \ = \ \frac{5x+328}{106}.\)

\(\displaystyle See \ graph.\)

[attachment=0:1gseb7b0]zero.jpg[/attachment:1gseb7b0]