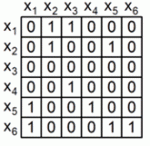
Please explain to me how to find a direct transitive closure for x2 on this adjacency matrix?
x1x2x3x4x5x6x1000011x2110000x3100100x4000010x5010001x6000001 . . . . . T+x2??????
I don't understand.
x1x2x3x4x5x6x1000011x2110000x3100100x4000010x5010001x6000001 . . . . . T+x2??????
I don't understand.
Attachments
Last edited by a moderator: