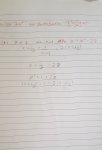Help w/ finding all solns of complex eqns: (z−i)^6=−64, z^2−z^(−2)=4i, z+z ^(−1)=2¯z
Ive been working on these for days but cant find any notes from my tutorials or lectures on how to solve them need help please!
I got a solutions to the first 2 but am in no way confident in saying they are correct!
a) Find all numbers z ∈ C such that (z − i)^6 = −64.
b)Find all z ∈ C such that z^2 − z^(−2) = 4i.
c) Find all z ∈ C such that z + z ^(−1) = 2¯z .
Ive been working on these for days but cant find any notes from my tutorials or lectures on how to solve them need help please!
I got a solutions to the first 2 but am in no way confident in saying they are correct!
a) Find all numbers z ∈ C such that (z − i)^6 = −64.
b)Find all z ∈ C such that z^2 − z^(−2) = 4i.
c) Find all z ∈ C such that z + z ^(−1) = 2¯z .