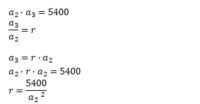Help regarding geometric progressions

W

Wilson123456789

New member
Joined
Aug 17, 2020
Messages
7
I have this exercise i've been having issues with for the last 3 days:
The result of the multiplication between the second and 3rd term of a geometric progression is 5400. What is the common ratio of this progression?
 
Please write an expression for the 2nd term of the geometric progression.
Please write an expression for the 3rd term of the geometric progression.
Please write an equation that represents the operation and result given in the problem statement.

Are we told ANYTHING else? Information seems a little sparse.
 
tkhunny said:
Please write an expression for the 2nd term of the geometric progression.
Please write an expression for the 3rd term of the geometric progression.
Please write an equation that represents the operation and result given in the problem statement.

Are we told ANYTHING else? Information seems a little sparse.
Click to expand...
I don't have extra information, that is my issue.
1597697014448.pngThis is what i've done so far, but idk how to continue
 

Attachments

  • 1597697006873.png
    1597697006873.png
    2.1 KB · Views: 3
Here's the plan -- put that in the FIRST POST. Don't make us guess. Thanks for keeping on top of it.

Maybe [math]a_{1}[/math] is an integer?

In other words: [math]a_{1}^{2}\cdot r^{3} = 5400[/math]
Did you notice that [math]5400 = 2^{3}\cdot 3^{3} \cdot 5^{2}[/math]? It's not clear that this does any good, but it is interesting.

Well, if [math]a_{1}[/math] is an integer, we now have a candidate. Of course, I just made up the extra requirement.
 
Wilson123456789 said:
I don't have extra information, that is my issue.
This is what i've done so far, but idk how to continue
Click to expand...
You must have been given the first term of the geometric series.
The first three terms look like: \(\large a,~ar,~\&~ar^2\)
Please review the statement for that first term.
 
tkhunny said:
Here's the plan -- put that in the FIRST POST. Don't make us guess. Thanks for keeping on top of it.

Maybe [math]a_{1}[/math] is an integer?

In other words: [math]a_{1}^{2}\cdot r^{3} = 5400[/math]
Did you notice that [math]5400 = 2^{3}\cdot 3^{3} \cdot 5^{2}[/math]? It's not clear that this does any good, but it is interesting.

Well, if [math]a_{1}[/math] is an integer, we now have a candidate. Of course, I just made up the extra requirement.
Click to expand...
Professor said it shouldn't need any other requirements, but i don't think that's right.
 
pka said:
You must have been given the first term of the geometric series.
The first three terms look like: \(\large a,~ar,~\&~ar^2\)
Please review the statement for that first term.
Click to expand...
I don't have any term at all, that's my issue.
 
Did you copy the entire problem as given to you? Is there anything said in context, such as in instructions for a set of exercises, that might be useful?

Clearly the problem as you've told us lacks information, so either there is something more, or it is just a bad problem and you can skip it.
 
Dr.Peterson said:
Did you copy the entire problem as given to you? Is there anything said in context, such as in instructions for a set of exercises, that might be useful?

Clearly the problem as you've told us lacks information, so either there is something more, or it is just a bad problem and you can skip it.
Click to expand...
Yes, i did. I do think the problem is missing data, thanks for reinforcing my thoughts, will ask that to the professor.
 
Re: spending days on a problem.

I do not think it is always bad. Maybe for lower level math it is not always good but...

I remember when I was a student studying Algebra from Herstein (Topics in Algebra). Our professor gave us 10 problems (all proofs) to do.

Maybe I could do a couple of them right away. Seven of them took me days to do but in the end I did them on my own. One of them I remembered seeing in a book. I found the proof in my book and after reading it I thought that the proof was somewhat obvious.

The day that the assignment was due I noticed that I had left it at home. I thought no problem and proceeded to write up the 10 proofs. Would you believe that I could only do 9 of them? The one that I copied from the book I could not remember how to do. I then went home retrieved the assignment and went to class. Of course while at home I looked at the proof. It was not difficult at all, yet I could not do it. In my opinion it was because I did not suffer enough to get the proof
 
You must log in or register to reply here.
Top