G
Guest
Guest
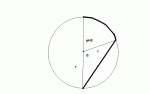
Suzie walks at 4 mph and rows at 2 mph. She wishes to get from a point on the edge of a circular pond to a point directly opposite. She can row to any point and walk along the edge the rest of the way. Find the fastest route/time.