logistic_guy
Senior Member
- Joined
- Apr 17, 2024
- Messages
- 1,610
here is the question
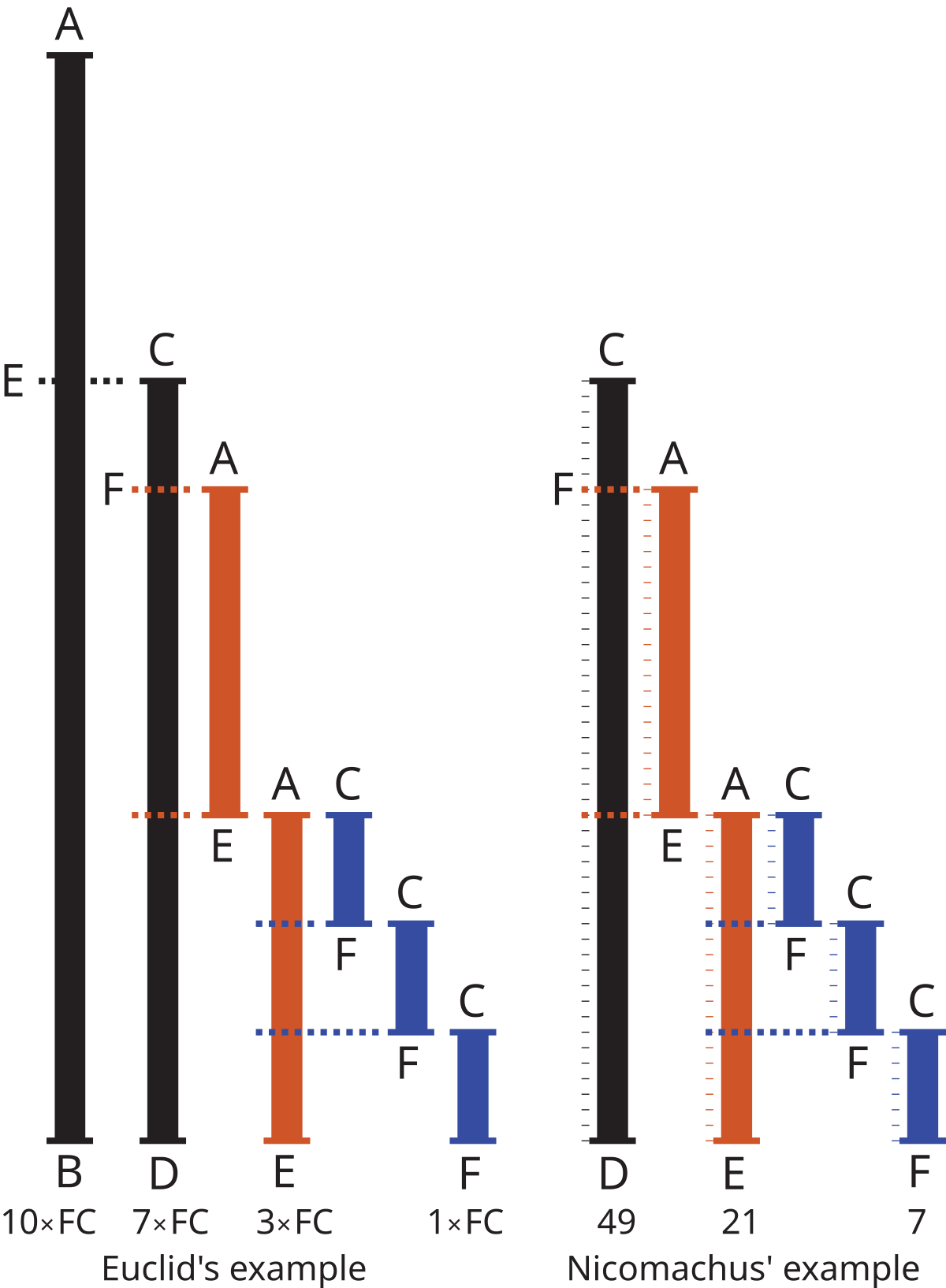
How to find the common greatest divisor of two large numbers, the first consists of 17 digits and the second consists of 16 digits?
it's easy when the numbers small like 50 and 90
50=2×5×5=2×52
90=2×3×3×5=2×32×5
gcd(50,90)=2×5=10
How to find the common greatest divisor of two large numbers, the first consists of 17 digits and the second consists of 16 digits?
it's easy when the numbers small like 50 and 90
50=2×5×5=2×52
90=2×3×3×5=2×32×5
gcd(50,90)=2×5=10