Yuseph
Junior Member
- Joined
- Aug 5, 2020
- Messages
- 68
Yo guys,
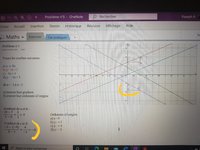
Its chapter 17 now. straight line graph.
Look, at first i thought the formula to get the gradient was (highest y point minus lowest y point) divided by (x point aligned with highest y point minus the other x point). But shortly after i did an exercice where the lowest y point came as the 1st element to be substracted.
At that point it became obvious that it wasnt about highest or lowest. It was about what you consider as being the front of the line.
But its confusing because a graph should.be read from left to right so im not sure why the author would make the gradient a number that indicate a backward evolution.
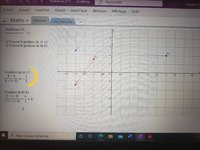
Its chapter 17 now. straight line graph.
Look, at first i thought the formula to get the gradient was (highest y point minus lowest y point) divided by (x point aligned with highest y point minus the other x point). But shortly after i did an exercice where the lowest y point came as the 1st element to be substracted.
At that point it became obvious that it wasnt about highest or lowest. It was about what you consider as being the front of the line.
But its confusing because a graph should.be read from left to right so im not sure why the author would make the gradient a number that indicate a backward evolution.