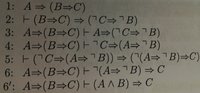Formal logic ?

[mar_f]

Hello, we had this in Mathematical Logic class: [MATH]A \implies (B \implies C)\vdash (A \wedge B) \implies C [/MATH]I have the solution for this but the problem is that I have no idea how to do it. I suppose that I have to show somehow that this formula [MATH](A \wedge B) \implies C[/MATH] is provable from this [MATH]A \implies (B \implies C)[/MATH]. We used here modus ponens, syllogism and some basic axioms, but I have no idea what does each of the steps mean and how to do that. The only thing I know is that I can rewrite the [MATH]A \wedge B[/MATH] using negation and implication.
Can somebody please explain me what is the main idea here?

Thanks for your help.

 

[pka]

This proof relies on two basic equivalences:
1) \(\displaystyle (X \Rightarrow Y) \equiv (\neg X \vee Y)\)
2) \(\displaystyle (\neg X \vee \neg Y) \equiv \neg (X \wedge Y)\)
Please show us how this applies to your question.