Fluid Force

[Brain0991]

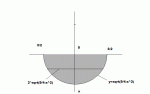
A cylindrical gasoline tank is placed so that the axis of the cylinder is horizontal. Find the fluid force on a circular end of the tank if the tank is half full of gas. Assume the diameter is 3 feet and gasoline weighs 42 pounds per cubic foot.

Can someone just get me started on this problem. I know theres going to be integrals in there somewhere.

 

[galactus]

The end of the tank is circular. Therein lies the crux of the matter.

The radius is 3/2.

Since it is half full, we integrate from0 to 3/2. Multiply by 2 because the entire circle has equation \(\displaystyle \pm\sqrt{r^{2}-x^{2}}\)

\(\displaystyle 42\int_{0}^{\frac{3}{2}}x\left(2\sqrt{\frac{9}{4}-x^{2}}\right)dx\)