How much work is done to bring a load of ore to the surface if a miner uses a cable weighing 2 lb/ft to haul a 100 lb bucket of ore up a mineshaft 800 feet deep?
ok so... any pointers on where to start?? i began using related rates techniques but that didnt account for the weight and such..
also this problem was given as a "challenge" problem so it's really got me confused..here are the teacher's instructions..
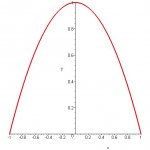
The base of a certain solid is a plane in region R enclosed by the x-axis and the curve y=1-x[sup:273p888g]2[/sup:273p888g]. Each cross-section of the solid perpendicular to the y-axis is an equilateral triangle with its base lying in R. Find the volume of the solid.
ok so... any pointers on where to start?? i began using related rates techniques but that didnt account for the weight and such..
also this problem was given as a "challenge" problem so it's really got me confused..here are the teacher's instructions..
The base of a certain solid is a plane in region R enclosed by the x-axis and the curve y=1-x[sup:273p888g]2[/sup:273p888g]. Each cross-section of the solid perpendicular to the y-axis is an equilateral triangle with its base lying in R. Find the volume of the solid.