It really is helpful to have a diagram.
You could learn a technique off by heart and apply it
if you can consistently remember the exact situations to use that technique in.
As i find that akin to reading in the dark,
i sincerely admire students who can do maths that way.
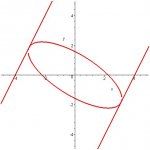
Maybe i'm lazy but here's my picture....[attachment=0:1pugyxxc]intersection.jpg[/attachment:1pugyxxc]
The red line is y=2x, or y=2x+0, a line with a slope of 2 and goes through the origin.
y=2x+k also has a slope of 2 but goes through the y axis at k
and there may be more than 1 possible value for k, depending on the curve in other cases,
but the lines will be parallel to y=2x.
Therefore, the lines intersect the curve at only 1 position,
they are tangents to the curve.
Therefore, where these lines touch the curve, we can say....
The (x,y) co-ordinates of the point of intersection are on both the line and curve.
For the red line there are two possible answers, corresponding to k=0,
but for the green ones, there is only one answer for each line,
but corresponding to two values for k.
In your exams, of course you cannot draw the curve unless you are imaginatively visual,
however the diagrams help you visualise what goes on between tangents and curves in general before you get there.
Based on the graph, we must solve for a single solution for the set of equations,
that is a single x and a single y, corresponding to the single point of intersection.
This then shows our options for k, and will discover both grren lines.
In contrast, all red lines between the two greens have 2 solutions for x and therefore 2 solutions for y,
but both of the green lines have only one solution each.
Therefore, if we take the y from the line and place it into the curve equation
and continue to solve for only 1 possible answer, we will find the k values for the 2 green lines.
\(\displaystyle y=2x+k\)
\(\displaystyle x^2+2xy+2y^2-5=0\)
therefore...
\(\displaystyle x^2+2x(2x+k)+2(2x+k)^2-5=0\)
Now finding how many 'x squared', how many 'x' and how many 'non-x' we have...
\(\displaystyle x^2+4x^2+2xk+2(2x+k)(2x+k)-5=0\)
\(\displaystyle x^2+4x^2+2xk+2(4x^2+2xk+2xk+k^2)-5=0\)
\(\displaystyle x^2+4x^2+2xk+8x^2+8xk+2k^2-5=0\)
\(\displaystyle (13)x^2+(10k)x+(2k^2-5)=0\)
Now we use the quadratic equation, (the abc robot) to find the values of x for which
a line of slope 2 intersects that curve....
\(\displaystyle ax^2+bx+c=0..........x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}\)
\(\displaystyle x=\frac{-10k\pm\sqrt{100k^2-4(13)(2k^2-5)}}{2(13}\)
We are specifically seeking the only one possible x answer...
There is only one answer when
\(\displaystyle 100k^2-4(13)(2k^2-5)=0\)
This now gives us the values of k (the y-axis crossing points) for which
a line of slope 2 is tangential to the curve.
Hence,
\(\displaystyle 100k^2=104k^2-260\)
\(\displaystyle 104k^2-100k^2=260\)
\(\displaystyle k^2=\frac{260}{4}=65\)
\(\displaystyle k=\pm\sqrt{65}\)