finding the tangents and normals

[shake123]

can you tell me a step-by step guide on how you work this question out.....

Find the normal to x² + y² - 4x - 30 = 0 at (7,3) ??

 

[galactus]

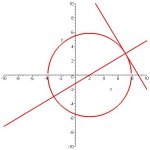
There are various ways to tackle it. This is a circle centered at (2,0) with radius sqrt(30).

\(\displaystyle x^{2}+y^{2}-4x=30\)

Differentiate implicitly:

\(\displaystyle 2x-2yy'-4=0\)

\(\displaystyle y'=\frac{4-2x}{2y}\)

Now, since x=7 and y=3, \(\displaystyle y'=\frac{-5}{3}\)

That is the slope of the tangent line at (7,3)

We want the normal. It's slope will be the negative reciprocal of that.

Then, use that slope, and the point (7,3) to find the equation of the line that is normal at that point.

Use y=mx+b. Plug them in and solve for b. That's it.

 

[BigGlenntheHeavy]

Here is the graph, can you figured it out?

[attachment=0:1o9uhams]abc.jpg[/attachment:1o9uhams]

 

[shake123]

Thanks a lot for the help! I appreciate it! x