Finding the limits of a polar curve

[MarkSA]

Hello,

I'm doing problems that involve finding the area that a polar curve encloses. I'm having trouble figuring out what limits to use when setting up the integral. My teacher basically set in class that the way to do it was to punch in common values like pi, pi/2, pi/4 etc for the limits to find which ones to use that draw the curve exactly once. This is sometimes taking a while though and seems like it could be inefficient, so i'm wondering if there is a better way to find the limits?

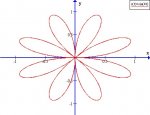
Example: given the curve: r = sin(4*theta). Through trial and area I came up with 0,pi/4 for the limits but it took a while and it's difficult to say from the graphing calculator if it's drawn exactly one curve and not repeated any.

 

[galactus]

Set your \(\displaystyle sin(4\theta})=0\). We find the solutions are \(\displaystyle \frac{\pi}{4}C\)

So, we can integrate from 0 to Pi/4. Since their are 8 petals to the rose, we multiply by 8.

\(\displaystyle 8\cdot\frac{1}{2}\int_{0}^{\frac{\pi}{4}}(sin(4\theta))^{2}d{\theta}\)

 

[MarkSA]

Thanks for the reply.

I'm unclear about one point. Is the reason that you set sin(4theta) = 0, because the origin is where the intersection points on the graph are?

 

[galactus]

Yep